Page 40 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 2: Legendre’s Polynomials

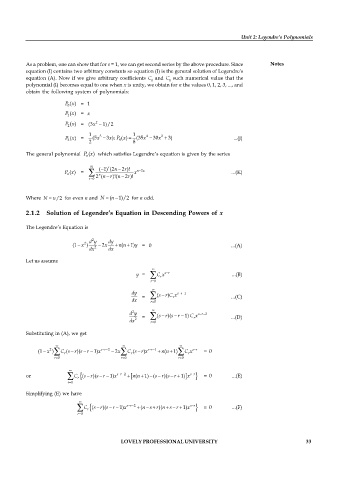
As a problem, one can show that for s = 1, we can get second series by the above procedure. Since Notes
equation (I) contains two arbitrary constants so equation (I) is the general solution of Legendre’s
equation (A). Now if we give arbitrary coefficients C and C such numerical value that the
0 1
polynomial (I) becomes equal to one when x is unity, we obtain for n the values 0, 1, 2, 3, ..., and
obtain the following system of polynomials:
x
P ( ) = 1
0
P 1 ( ) = x
x
x
P 2 ( ) = (3x 2 1)/2
1 3 1 4 2
x
P 3 ( ) = (5x 3 ); P 4 ( ) (35x 30x 3) ...(J)
x
x
2 8
The general polynomial P n ( ) which satisfies Legendre’s equation is given by the series
x
N ( 1) (2n 2 )!
r
r
x
P n ( ) = n x n 2x ...(K)
r
r 0 2 (n r )!(n 2 )!
Where N n /2 for even n and N (n 1)/2 for n odd.
2.1.2 Solution of Legendre’s Equation in Descending Powers of x

The Legendre’s Equation is

2
2 d y dy
(1 x ) 2x ( n n 1)y = 0 ...(A)
dx 2 dx
Let us assume

y = C x s r ...(B)
r
r 0
dy s r 1
= (s r )C x ...(C)
r
dx r 0
2
d y s r 2
= (s r )(s r 1) C x ...(D)
r
dx 2 r 0
Substituting in (A), we get

(1 x 2 ) C r (s r )(s r 1)x s r 2 2x C r (s r )x s r 1 ( n n 1) C x s r = 0
r
r 0 r 0 r 0

or C r (s r )(s r 1)x s r 2 ( n n 1) (s r )(s r 1) x s r = 0 ...(E)
r 0
Simplifying (E) we have

C r (s r )(s r 1)x s r 2 (n s r )(n s r 1)x s r 0 ...(F)
r 0

LOVELY PROFESSIONAL UNIVERSITY 33