Page 75 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes Self Assessment

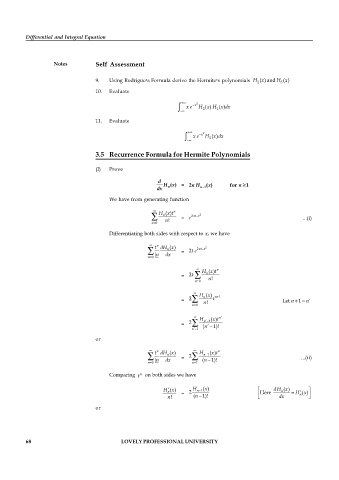
x
9. Using Rodrigue s Formula derive the Hermite s polynomials H 2 ( ) and H 3 ( )
x
10. Evaluate
2
x
x
x
x e H 2 ( ) H 1 ( )dx
11. Evaluate
x
x e x 2 H 2 ( )dx

3.5 Recurrence Formula for Hermite Polynomials

(I) Prove
d
H n ( ) = 2n H n 1 ( ) for n 1
x
x
dx
We have from generating function
H n ( )t n 2xt t 2
x
! n = e ...(i)
n 0
Differentiating both sides with respect to x, we have

n
x
t dH n ( ) 2t e 2xt t 2
n dx =
n 0
x
H n ( )t n
= 2t ! n
n 0
H x 1
( ) n
n
= 2 ! n t Let n 1 n
n 0
H n 1 ( )t n
x
= 2 n 1 !
n 1
or

n
t dH ( ) H ( )t n
x
x
n 2 n 1
n dx = (n 1)! ...(ii)
n 0 n 1
n
Comparing t on both sides we have
x
x
x
H n ( ) = 2 H n 1 ( ) Here dH n ( ) H ( )
x
! n (n 1)! dx n
or

68 LOVELY PROFESSIONAL UNIVERSITY