Page 97 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes
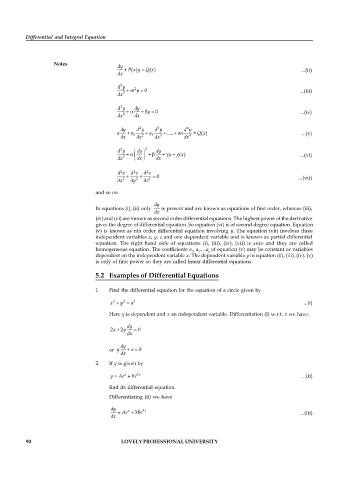
dy
P ( )y Q ( ) ...(ii)
x
x
dx
2
d y 2
m y 0 ...(iii)
dx 2
2
d y dy y 0
dx 2 dx ...(iv)
2
n
3
dy d y d y d y
x
a a 2 a 3 ..... an Q ( ) ...(v)
dx dx 2 dx 3 dx n
2
d y dy 2 dy y g ( )
x
dx 2 dx dx ...(vi)
2 2 2
v v v
0 ...(vii)
x 2 y 2 z 2
and so on.
dy
In equations (i), (ii) only is present and are known as equations of first order, whereas (iii),
dx
(iv) and (vi) are known as second order differential equations. The highest power of the derivative
gives the degree of differential equation. So equation (vi) is of second degree equation. Equation
(v) is known as nth order differential equation involving y. The equation (vii) involves three
independent variables x, y, z and one dependent variable and is known as partial differential
equation. The right hand side of equations (i), (iii), (iv), (vii) is zero and they are called
homogeneous equation. The coefficients a , a .....a of equation (v) may be constant or variables
1 2 n
dependent on the independent variable x. The dependent variable y in equation (ii), (iii), (iv), (v)
is only of first power so they are called linear differential equations.

5.2 Examples of Differential Equations

1. Find the differential equation for the equation of a circle given by

x 2 y 2 a 2 ...(i)
Here y is dependent and x an independent variable. Differentiation (I) w.r.t. x we have:

dy
2x 2y 0
dx
dy
or y x 0
dx
2. If y is given by

y Ae x Be 3x ...(ii)
find its differential equation.
Differentiating (ii) we have

dy x 3x
Ae 3Be ...(iii)
dx

90 LOVELY PROFESSIONAL UNIVERSITY