Page 101 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

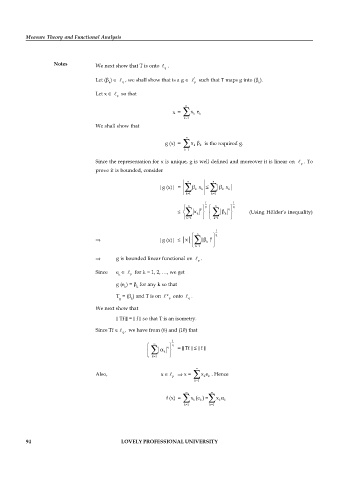
Notes We next show that T is onto  .
q
*
Let ( )  , we shall show that is a g  such that T maps g into ( ).
k q p k
Let x  so that
p
n
x = x e k
k
k 1
We shall show that

n
g (x) = x k k is the required g.
k 1

Since the representation for x is unique, g is well defined and moreover it is linear on  . To
p
prove it is bounded, consider

n n
|g (x)| = k x k k x k
k 1 k 1
1 1
n p n q
p q
x k k (Using Hölder’s inequality)
k 1 k 1
1
n q
|g (x)| x | | q
k
k 1
g is bounded linear functional on  .
p
Since e  for k = 1, 2, …, we get
k p
g (e ) = for any k so that
k k
T = ( ) and T is on  * onto  .
g k p q
We next show that
Tf = f so that T is an isometry.

Since Tf  , we have from (6) and (10) that
q
1
q
q = Tf f
k
k 1

Also, x  x = x e k . Hence
p k
k 1

f (x) = x (e ) x k k
k
k
k 1 k 1

94 LOVELY PROFESSIONAL UNIVERSITY