Page 97 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

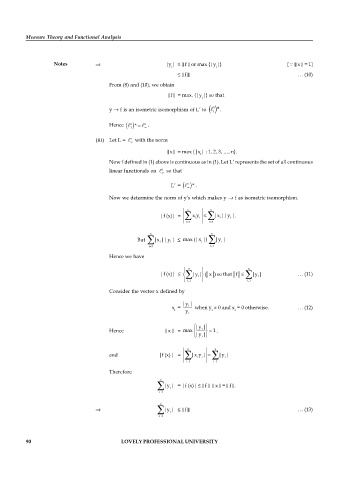
Notes |y | f or max {|y |} [ x = 1]
i i
f … (10)
From (8) and (10), we obtain
f = max. {|y |} so that
i
n
y f is an isometric isomorphism of L to  *.
1
n
n
Hence  *  .
1
n
(iii) Let L =  with the norm
x = max {|x | : 1, 2, 3, …, n}.
i
Now f defined in (1) above is continuous as in (1). Let L represents the set of all continuous
n
linear functionals on  so that
L =  n * .

Now we determine the norm of y’s which makes y f as isometric isomorphism.

n n
|f (x)| = x y i |x ||y |.
i
i
i
i 1 i 1
n n
But |x ||y | max (|x |) |y |
i i i i
i 1 i 1
Hence we have

n n
|f (x)| |y | ( x ) so that f |y | … (11)
i
i
i 1 i 1
Consider the vector x defined by
|y |
x = i when y 0 and x = 0 otherwise. … (12)
i y i i
i
|y |
Hence x = max i 1 .
|y |
i
n n
and |f (x)| = |x y | |y |
i i i
i 1 i 1
Therefore
n
|y | = |f (x)| f x = f .
i
i 1
n
|y | f … (13)
i
i 1

90 LOVELY PROFESSIONAL UNIVERSITY