Page 11 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

Notes
It also I I = , we should have  I  . Further if the interval I does not meet any of the
N+1 N 1
intervals in the sequence <I >, we must have
r
 I  , r
r
which is not true as  2 (I ) 0 as r .

r r 1
I must meet at least one of the intervals of the sequence <I >. Let p be the least integer s.t. I
r
meets I . Then p > N and (I)   2 (I ). Further let x I as well x I , then the distance of x
p p 1 p p
from the mid point of I is at most
p
1 1 5

 (I) (   ) 2 (I )  (I )  (I )
2 p p 2 p 2 p
Thus if I is an interval having the same mid point as I but length 5 times the length of I , i.e.
p p p
(J ) 5 (I ) . Then x J also.
p p p
Thus for every x F, an integer p > N s.t. x J
p
and (J ) 5 (I ) . Also
p p

F J p
p N 1

m *(F) (J ) 5 (I ) 5
p p 5
p N 1 p N 1
and hence the Lemma holds good.

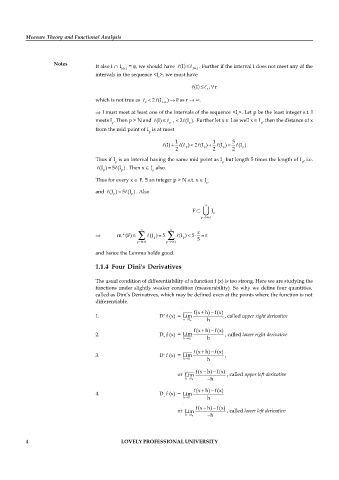
1.1.4 Four Dini's Derivatives

The usual condition of differentiability of a function f (x) is too strong. Here we are studying the
functions under slightly weaker condition (measurability). So why we define four quantities,
called as Dini’s Derivatives, which may be defined even at the points where the function is not
differentiable.
f(x h) f(x)
+
1. D f (x) = Lim , called upper right derivative
n 0 h
f(x h) f(x)
2. D f (x) = Lim , called lower right derivative
+ h 0 h

f(x h) f(x)
3. D f (x) = Lim ,
–
h 0 h
f(x h) f(x)
or Lim , called upper left derivative
h 0 h
f(x h) f(x)
4. D f (x) = Lim
–
h 0 h
f(x h) f(x)
or Lim , called lower left derivative
h 0 h

4 LOVELY PROFESSIONAL UNIVERSITY