Page 130 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 11: Integration

Notes
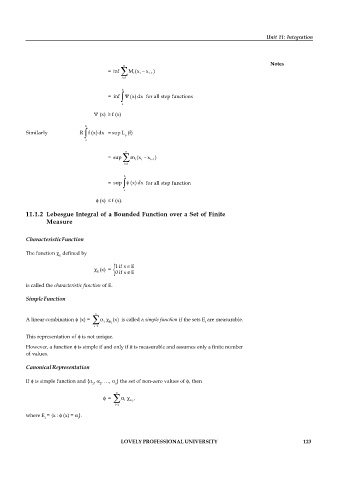
n
= inf M (x i x )
i 1
i
i 1
b
= inf (x) dx for all step functions
a

(x) f (x)
b
Similarly R f (x) dx = sup L (f)
p
a
n
= sup m (x i x )
i 1
i
i 1
b
= sup (x) dx for all step function
a

(x) f (x).
11.1.2 Lebesgue Integral of a Bounded Function over a Set of Finite
Measure

Characteristic Function

The function defined by
E
1 if x E
(x) =
E 0 if x E
is called the characteristic function of E.

Simple Function

n
A linear combination (x) = (x) is called a simple function if the sets E are measurable.
i E i i
i 1
This representation of is not unique.

However, a function is simple if and only if it is measurable and assumes only a finite number
of values.

Canonical Representation

If is simple function and { , , …, } the set of non-zero values of , then
1 2 n
n
= ,
i E i
i 1
where E = {x : (x) = }.
i i

LOVELY PROFESSIONAL UNIVERSITY 123