Page 135 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

Notes
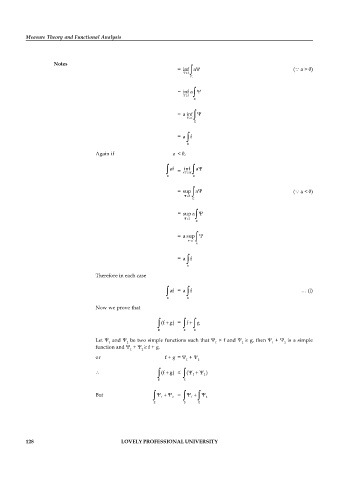
= inf a ( a > 0)
f
E

= inf a
f
E

= a inf
f
E

= a f
E
Again if a < 0,

af inf a
= a af
E E

= sup a ( a < 0)
f
E
= sup a
f
E
= a sup
f
E
= a f
E
Therefore in each case

af = a f … (i)
E E
Now we prove that

(f g) = f g
E E E
Let and be two simple functions such that > f and g, then + is a simple
1 2 1 2 1 2
function and + f + g.
1 2
or f + g = +
1 2
(f g) ( )
1 2
E E

But =
1 2 1 2
E E E

128 LOVELY PROFESSIONAL UNIVERSITY