Page 137 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

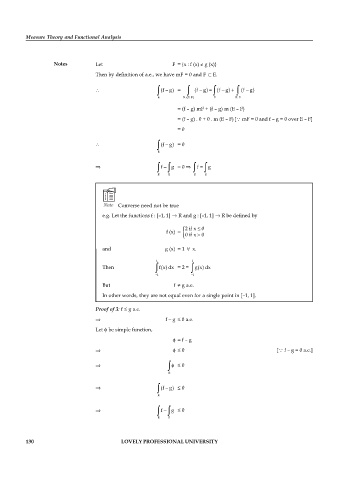
Notes Let F = {x : f (x) g (x)}
Then by definition of a.e., we have mF = 0 and F E.

(f g) = (f g) (f g) (f g)
E F (E F) F E F
= (f – g) mF + (f – g) m (E – F)

= (f – g) . 0 + 0 . m (E – F) [ mF = 0 and f – g = 0 over E – F]
= 0

(f g) = 0
E

f g = 0 f g
E E E E

Note Converse need not be true
e.g. Let the functions f : [–1, 1] R and g : [–1, 1] R be defined by

2 if x 0
f (x) =
0 if x 0
and g (x) = 1 x.

1 1
Then f(x) dx = 2 = g(x) dx
1 1
But f g a.e.
In other words, they are not equal even for a single point in [–1, 1].

Proof of 3: f g a.e.

f – g 0 a.e.
Let be simple function,
= f – g
0 [ f – g = 0 a.e.]

0
E

(f g) 0
E

f g 0
E E

130 LOVELY PROFESSIONAL UNIVERSITY