Page 132 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 11: Integration

Notes
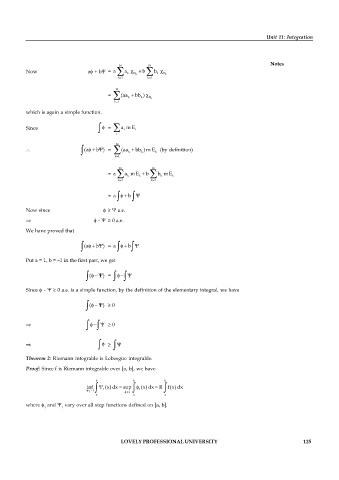
N N
Now a + b = a a k E k b b k E k
k 1 k 1
N
= (aa k bb ) E k
k
k 1
which is again a simple function.

Since = a m E i
i
i
N
(a b ) = (aa bb ) m E (by definition)
k k k
k 1

N N
= a a mE k b b mE k
k
k
k 1 k 1
= a b

Now since a.e.
– 0 a.e.
We have proved that

(a b ) = a b

Put a = 1, b = –1 in the first part, we get

( ) =

Since – 0 a.e. is a simple function, by the definition of the elementary integral, we have

( ) 0

0

Theorem 2: Riemann integrable is Lebesgue integrable.
Proof: Since f is Riemann integrable over [a, b], we have

b b b
inf 1 (x) dx sup 1 (x) dx R f(x) dx
1 f f
a a a
where and vary over all step functions defined on [a, b].
1 1

LOVELY PROFESSIONAL UNIVERSITY 125