Page 133 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

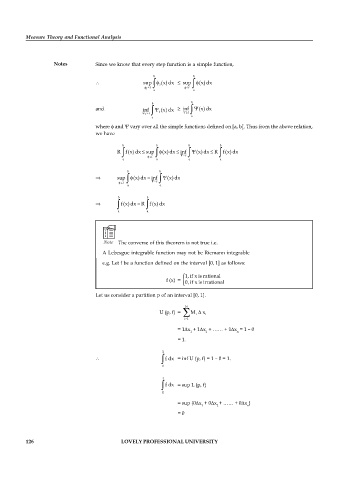
Notes Since we know that every step function is a simple function,
b b
sup (x) dx sup (x) dx
1
1 f f
a a
b b
and inf (x) dx inf (x) dx
1 f 1 f
a a
where and vary over all the simple functions defined on [a, b]. Thus from the above relation,
we have

b b b b
R f(x) dx sup (x) dx inf (x) dx R f(x) dx
f
f
a a a a
b b
sup (x) dx inf (x) dx
f f
a a
b b
f(x) dx R f(x) dx
a a

Note The converse of this theorem is not true i.e.
A Lebesgue integrable function may not be Riemann integrable
e.g. Let f be a function defined on the interval [0, 1] as follows:

1, if x is rational
f (x) =
0, if x is irrational
Let us consider a partition p of an interval [0, 1].

N
U (p, f) = M x
i i
i 1
= 1 x + 1 x + …… + 1 x = 1 – 0
1 2 n
= 1.

1
f dx = inf U (p, f) = 1 – 0 = 1.
0

1
f dx = sup L (p, f)
0
= sup {0 x + 0 x + …… + 0 x }
1 2 n
= 0

126 LOVELY PROFESSIONAL UNIVERSITY