Page 198 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 16: Continuous Linear Transformations

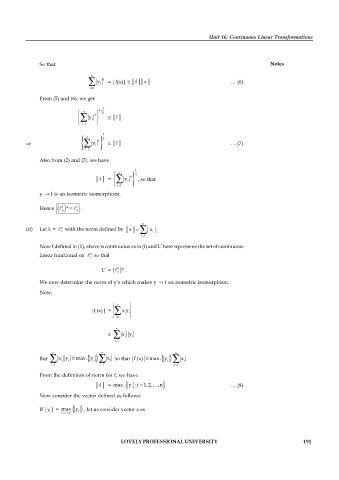
So that Notes
n
q
y i = |f(x)| f x … (6)
i 1
From (5) and (6), we get

1
n 1 p
q
y f
i
i 1
1
n q
q
y f … (7)
i
i 1
Also from (2) and (7), we have
1
n q
q
f = y i , so that
i 1
y f is an isometric isomorphism.

Hence  n *  n q .
p

n
n
(ii) Let L =  with the norm defined by x x .
1
i
i 1
Now f defined in (1), above is continuous as in (i) and L here represents the set of continuous
n
linear functional on  so that
1
L =  n * .
1
We now determine the norm of y’s which makes y f an isometric isomorphism.

Now,
n
|f (x)| = x y
i i
i 1
n
x y i
i
i 1
n n n
But x y i max. y i x i so that f(x) max. y i x .
i
i
i 1 i 1 i 1
From the definition of norm for f, we have
f = max. y : i 1,2, ,n … (8)
i
Now consider the vector defined as follows:

If |y | = max y i , let us consider vector x as
i 1 i n

LOVELY PROFESSIONAL UNIVERSITY 191