Page 227 - DSOC404_METHODOLOGY_OF_SOCIAL_RESEARCH_ENGLISH

Unit–28: Measures of Dispersion: Standard Deviation

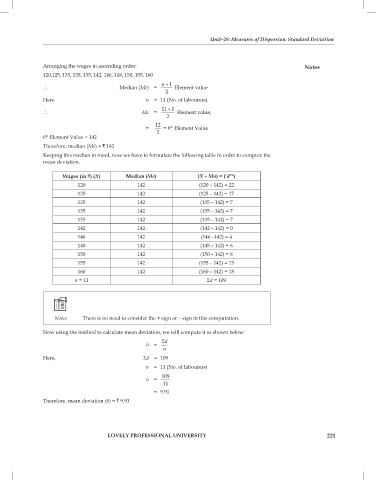
Arranging the wages in ascending order: notes
120,125, 135, 135, 135, 142, 146, 148, 150, 155, 160
n + 1
\ Median (Me) = element value
2
Here n = 11 (No. of labourers)
11 1
+
\ Me = Element value;
2
12
= = 6 Element Value
th
2
6 Element Value = 142
th
therefore, median (Me) = ` 142
Keeping this median in mind, now we have to formulate the following table in order to compute the
mean deviation.

Wages (in `) (X) Median (Me) (X – Mo) = (‘d’*)
120 142 (120 – 142) = 22
125 142 (125 – 142) = 17
135 142 (135 – 142) = 7
135 142 (135 – 142) = 7
135 142 (135 – 142) = 7
142 142 (142 – 142) = 0
146 142 (146 –142) = 4
148 142 (148 – 142) = 6
150 142 (150 – 142) = 8
155 142 (155 – 142) = 13
160 142 (160 – 142) = 18
n = 11 Sd = 109

Notes there is no need to consider the + sign or – sign in this computation.

Now using the method to calculate mean deviation, we will compute it as shown below:
S d
d =
n
Here, Sd = 109
n = 11 (No. of labourers)
109
d =
11
= 9.91
therefore, mean deviation (d) = ` 9.91

loVely professional uniVersity 221