Page 208 - DMGT209_QUANTITATIVE_TECHNIQUES_II

Unit 10: Correlation

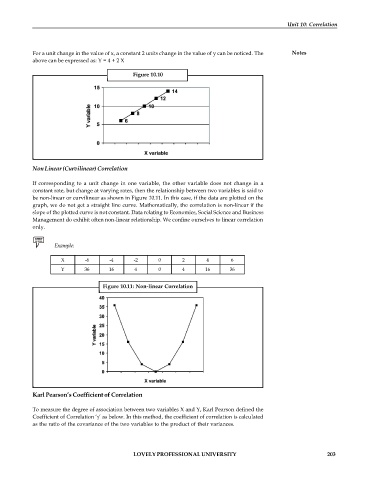
For a unit change in the value of x, a constant 2 units change in the value of y can be noticed. The Notes
above can be expressed as: Y = 4 + 2 X

Figure 10.10

Non Linear (Curvilinear) Correlation

If corresponding to a unit change in one variable, the other variable does not change in a
constant rate, but change at varying rates, then the relationship between two variables is said to
be non-linear or curvilinear as shown in Figure 10.11. In this case, if the data are plotted on the
graph, we do not get a straight line curve. Mathematically, the correlation is non-linear if the
slope of the plotted curve is not constant. Data relating to Economics, Social Science and Business
Management do exhibit often non-linear relationship. We confine ourselves to linear correlation
only.

Example:

X -6 -4 -2 0 2 4 6
Y 36 16 4 0 4 16 36

Figure 10.11: Non-linear Correlation

Karl Pearson’s Coefficient of Correlation

To measure the degree of association between two variables X and Y, Karl Pearson defined the
Coefficient of Correlation ‘’ as below. In this method, the coefficient of correlation is calculated
as the ratio of the covariance of the two variables to the product of their variances.

LOVELY PROFESSIONAL UNIVERSITY 203