Page 211 - DMGT209_QUANTITATIVE_TECHNIQUES_II
P. 211
Quantitative Techniques-II
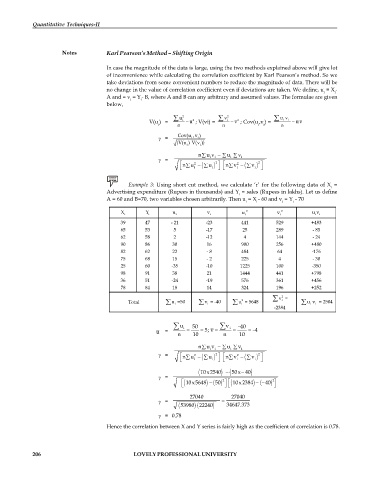
Notes Karl Pearson’s Method – Shifting Origin
In case the magnitude of the data is large, using the two methods explained above will give lot
of inconvenience while calculating the correlation coefficient by Karl Pearson’s method. So we
take deviations from some convenient numbers to reduce the magnitude of data. There will be
no change in the value of correlation coefficient even if deviations are taken. We define, u = X -
i i
A and = v = Y - B, where A and B can any arbitrary and assumed values. The formulae are given
i i
below,
2 i u 2 i v i u v
2
2
V(u ) = u ; V(vi) = v ; Cov(u ,v ) = i u v
i n n i i n
Cov(u ,v )
= i i
{V(u ) V(v )}
i i
n u v u i v i
i
i
=
2
2
n u 2 n v 2
u
v
i i i i
Example 3: Using short cut method, we calculate ‘r’ for the following data of X =
i
Advertising expenditure (Rupees in thousands) and Y = sales (Rupees in lakhs). Let us define
i
A = 60 and B=70, two variables chosen arbitrarily. Then u = X - 60 and v = Y - 70
i i i i
2
2
X Y u v i u i v u v
i
i
i
i
i
i
39 47 - 21 -23 441 529 +483
65 53 5 -17 25 289 - 85
62 58 2 -12 4 144 - 24
90 86 30 16 900 256 +480
82 62 22 - 8 484 64 -176
75 68 15 - 2 225 4 - 30
25 60 -35 -10 1225 100 -350
98 91 38 21 1444 441 +798
36 51 -24 -19 576 361 +456
78 84 18 14 324 196 +252
2
v =
2
Total u =50 v = -40 u = 5648 i u v = 2504
i
i
i
i
i
-2384
u i 50 v i 40
u = 5; v 4
n 10 n 10
n u v u i v i
i
i
= n u 2 n v 2
2
2
u
v
i i i i
10x2540 50x 40
=
2
10x5648 50 2 10x2384 40
27040 27040
=
53980 22240 34647.373
= 0.78
Hence the correlation between X and Y series is fairly high as the coefficient of correlation is 0.78.
206 LOVELY PROFESSIONAL UNIVERSITY