Page 29 - DMGT405_FINANCIAL%20MANAGEMENT

Unit 2: Time Value of Money

If the company needs a total of 3,00,000 on June 30, 2010, how much would it have to deposit Notes
every year? Here we have to solve for the rent, given the future value, as follows:
FV = Rent × f (n =5, i =11%)
3,00,000 = Rent × 6.22780

Rent = 3,00,000/6.22780 = 48,171.10
The company has to deposit 48,171 each time in order to accumulate the necessary 3,00,0000
by June 30, 2010.

2.3.2 Present Value of Annuity of 1

The present value of an annuity is the sum that must be invested today at compound interest in
order to obtain periodic rents over some future time.

Notice that we use the abbreviation PV for the present value of an annuity, as differentiated
from the lower case pv for the present value of 1. By using the present value of 1, we can obtain
a table for the present value of an ordinary annuity of 1. The present value of an ordinary
annuity of 1 can be illustrated as follows:
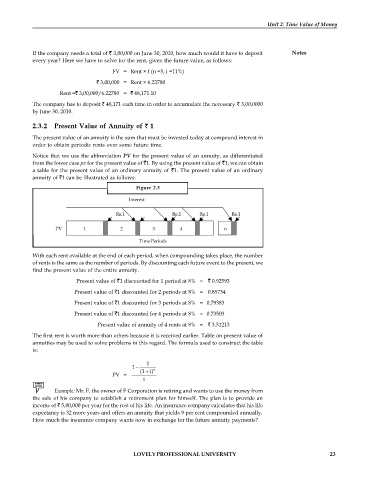
Figure 2.3

With each rent available at the end of each period, when compounding takes place, the number
of rents is the same as the number of periods. By discounting each future event to the present, we
find the present value of the entire annuity.

Present value of 1 discounted for 1 period at 8% = 0.92593
Present value of 1 discounted for 2 periods at 8% = 0.85734
Present value of 1 discounted for 3 periods at 8% = 0.79383
Present value of 1 discounted for 4 periods at 8% = 0.73503
Present value of annuity of 4 rents at 8% = 3.31213

The first rent is worth more than others because it is received earlier. Table on present value of
annuities may be used to solve problems in this regard. The formula used to construct the table
is:
1
1 -
(1 + i) n
PV =
i
Example: Mr. F, the owner of F Corporation is retiring and wants to use the money from
the sale of his company to establish a retirement plan for himself. The plan is to provide an
income of 5,00,000 per year for the rest of his life. An insurance company calculates that his life
expectancy is 32 more years and offers an annuity that yields 9 per cent compounded annually.
How much the insurance company wants now in exchange for the future annuity payments?

LOVELY PROFESSIONAL UNIVERSITY 23