Page 77 - DMGT405_FINANCIAL%20MANAGEMENT

Unit 5: Risk and Return Analysis

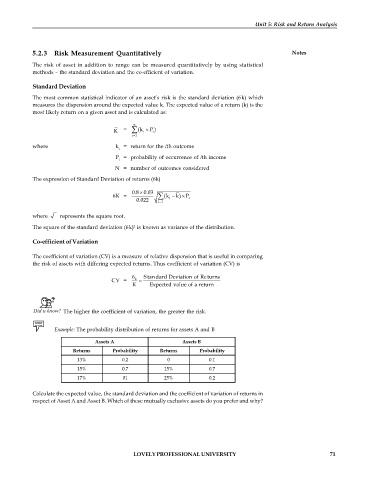
5.2.3 Risk Measurement Quantitatively Notes

The risk of asset in addition to range can be measured quantitatively by using statistical
methods – the standard deviation and the co-efficient of variation.

Standard Deviation

The most common statistical indicator of an asset’s risk is the standard deviation (6k) which
measures the dispension around the expected value k. The expected value of a return (k) is the
most likely return on a given asset and is calculated as:
n
K = å (k ´ P )
i
i
i 1
=
where k = return for the ith outcome
i
P = probability of occurrence of ith income
i
N = number of outcomes considered
The expression of Standard Deviation of returns (6k)

0.8 ´ 0.03
6K = å (k i - k) P i
´
0.022 = i 1
where represents the square root.
The square of the standard deviation (6k) is known as variance of the distribution.
2
Co-efficient of Variation

The coefficient of variation (CV) is a measure of relative dispension that is useful in comparing
the risk of assets with differing expected returns. Thus coefficient of variation (CV) is

6 Standard Deviation of Returns
CV = k =
K Expected value of a return

Did u know? The higher the coefficient of variation, the greater the risk.

Example: The probability distribution of returns for assets A and B
Assets A Assets B
Returns Probability Returns Probability
13% 0.2 0 0.1
15% 0.7 15% 0.7
17% 01 25% 0.2

Calculate the expected value, the standard deviation and the coefficient of variation of returns in
respect of Asset A and Asset B. Which of these mutually exclusive assets do you prefer and why?

LOVELY PROFESSIONAL UNIVERSITY 71