Page 81 - DMGT405_FINANCIAL%20MANAGEMENT

Unit 5: Risk and Return Analysis

5.3.2 Measuring Portfolio Risk Notes

Like in the case of individual assets or securities, the risk of a portfolio can be measured in terms
of variance or standard deviation. The portfolio variance is affected by the association of
movement of returns of two securities. Covariance of two securities measures their co-
movements. Three steps are involved in the calculation of covariance between two securities:

1. Determine the expected returns for securities.
2. Determine the deviation of possible returns for each security.
3. Determine the sum of the product of each deviation of returns of two securities and
probability.

!
Caution The variance or standard deviation of the portfolio is not simply the weighted
average of variances or standard deviations of individual securities.
Let us consider the data of securities X Y given in Example 4.
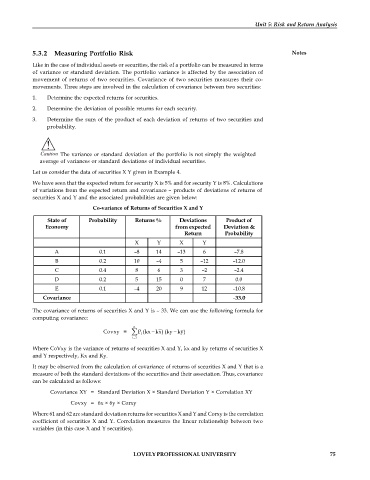
We have seen that the expected return for security X is 5% and for security Y is 8%. Calculations
of variations from the expected return and covariance – products of deviations of returns of
securities X and Y and the associated probabilities are given below:
Co-variance of Returns of Securities X and Y

State of Probability Returns % Deviations Product of
Economy from expected Deviation &
Return Probability
X Y X Y
A 0.1 –8 14 –13 6 –7.8
B 0.2 10 –4 5 –12 –12.0
C 0.4 8 6 3 –2 –2.4
D 0.2 5 15 0 7 0.0
E 0.1 –4 20 9 12 –10.8
Covariance –33.0

The covariance of returns of securities X and Y is – 33. We can use the following formula for
computing covariance:
n
Covxy =  P (kx kx) (ky ky)


1
i 1

Where CoVxy is the variance of returns of securities X and Y, kx and ky returns of securities X
and Y respectively, Kx and Ky.
It may be observed from the calculation of covariance of returns of securities X and Y that is a
measure of both the standard deviations of the securities and their association. Thus, covariance
can be calculated as follows:
Covariance XY = Standard Deviation X × Standard Deviation Y × Correlation XY

Covxy = 6x × 6y × Corxy
Where 61 and 62 are standard deviation returns for securities X and Y and Corxy is the correlation
coefficient of securities X and Y. Correlation measures the linear relationship between two
variables (in this case X and Y securities).

LOVELY PROFESSIONAL UNIVERSITY 75