Page 329 - DCOM504_SECURITY_ANALYSIS_AND_PORTFOLIO_MANAGEMENT

Security Analysis and Portfolio Management

Notes
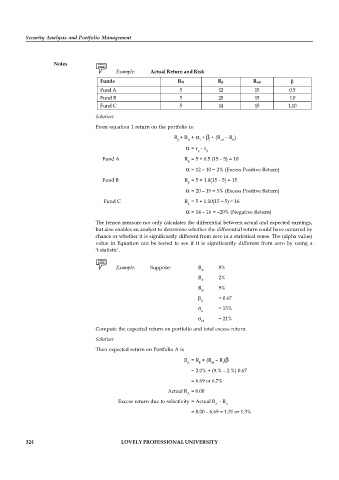
Example: Actual Return and Risk
Funds R ft R jt R mt
Fund A 5 12 15 0.5
Fund B 5 20 15 1.0
Fund C 5 14 15 1.10

Solution:
From equation 1 return on the portfolio is:

R + R + + + (R – R )
jt ft 1 j mt ft
= r – r
p jt
Fund A R = 5 + 0.5 (15 – 5) = 10
jt
= 12 – 10 = 2% (Excess Positive Return)
Fund B R = 5 + 1.0(15 – 5) = 15
jt
= 20 – 15 = 5% (Excess Positive Return)

Fund C R = 5 + 1.10(15 – 5) = 16
jt
= 14 – 16 = –20% (Negative Return)
The Jensen measure not only calculates the differential between actual and expected earnings,
but also enables an analyst to determine whether the differential return could have occurred by
chance or whether it is significantly different from zero in a statistical sense. The (alpha value)
value in Equation can be tested to see if it is significantly different from zero by using a
‘t statistic’.

Example: Suppose: R 8%
A
R 2%
F
R 9%
m
= 0.67
A
= 15%
A
= 21%
M
Compute the expected return on portfolio and total excess return.
Solution:
Then expected return on Portfolio A is
R = R + (R – R )
A F M J
= 2.0% + (9.% – 2.%) 0.67
= 6.69 or 6.7%
Actual R = 8.00
A
Excess return due to selectivity = Actual R – R
A A
= 8.00 – 6.69 = 1.31 or 1.3%

324 LOVELY PROFESSIONAL UNIVERSITY