Page 87 - DCOM510_FINANCIAL_DERIVATIVES

Financial Derivatives

Notes
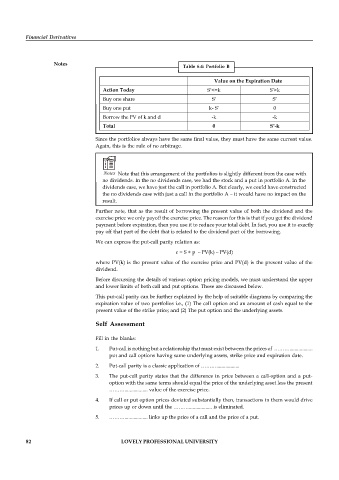
Table 6.4: Portfolio B
Value on the Expiration Date
*
*
Action Today S <=k S >k
Buy one share S S *
*
*
Buy one put k- S 0
Borrow the PV of k and d -k -k
Total 0 S -k
*

Since the portfolios always have the same final value, they must have the same current value.
Again, this is the rule of no arbitrage.

Notes Note that this arrangement of the portfolios is slightly different from the case with
no dividends. In the no dividends case, we had the stock and a put in portfolio A. In the
dividends case, we have just the call in portfolio A. But clearly, we could have constructed
the no dividends case with just a call in the portfolio A – it would have no impact on the
result.
Further note, that as the result of borrowing the present value of both the dividend and the
exercise price we only payoff the exercise price. The reason for this is that if you get the dividend
payment before expiration, then you use it to reduce your total debt. In fact, you use it to exactly
pay off that part of the debt that is related to the dividend part of the borrowing.

We can express the put-call parity relation as:
c = S + p – PV(k) – PV(d)
where PV(k) is the present value of the exercise price and PV(d) is the present value of the
dividend.
Before discussing the details of various option pricing models, we must understand the upper
and lower limits of both call and put options. These are discussed below.

This put-call parity can be further explained by the help of suitable diagrams by comparing the
expiration value of two portfolios i.e., (1) The call option and an amount of cash equal to the
present value of the strike price; and (2) The put option and the underlying assets.
Self Assessment

Fill in the blanks:
1. Put-call is nothing but a relationship that must exist between the prices of ………..................
put and call options having same underlying assets, strike price and expiration date.
2. Put-call parity is a classic application of ………..................
3. The put-call parity states that the difference in price between a call-option and a put-
option with the same terms should equal the price of the underlying asset less the present
……….................. value of the exercise price.
4. If call or put option prices deviated substantially then, transactions in them would drive
prices up or down until the ……….................. is eliminated.
5. ……….................. links up the price of a call and the price of a put.

82 LOVELY PROFESSIONAL UNIVERSITY