Page 92 - DCOM510_FINANCIAL_DERIVATIVES

Unit 6: Valuation and Pricing of Options

Notes
Did u know? Monte Carlo option models are generally used in these cases. Monte Carlo
simulation is, however, time-consuming in terms of computation.
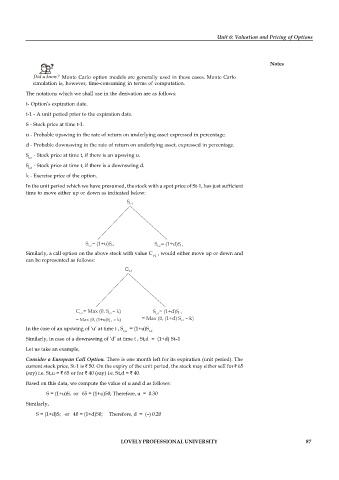
The notations which we shall use in the derivation are as follows:
t- Option’s expiration date.
t-1 - A unit period prior to the expiration date.

S - Stock price at time t-1.
u - Probable upswing in the rate of return on underlying asset expressed in percentage.
d - Probable downswing in the rate of return on underlying asset, expressed in percentage.

S - Stock price at time t, if there is an upswing u.
t,u
S - Stock price at time t, if there is a downswing d.
t,d
k - Exercise price of the option.
In the unit period which we have presumed, the stock with a spot price of St-1, has just sufficient
time to move either up or down as indicated below:
S t–1

t,u t,d
S = (1+u)S t–1 S = (1+d)S t–1
Similarly, a call option on the above stock with value C , would either move up or down and
t–1
can be represented as follows:
C t–1

C = Max (0, S – k) S = (1+d)S t–1
t,u
t,u
t,d
= Max (0, (1+u)S – k) = Max (0, (1+d) S – k)
t-1
t-1
In the case of an upswing of ‘u’ at time t , S = (1+u)S
t,u t–1
Similarly, in case of a downswing of ‘d’ at time t , St,d = (1+d) St–1
Let us take an example,
Consider a European Call Option. There is one month left for its expiration (unit period). The
current stock price, St-1 is ` 50. On the expiry of the unit period, the stock may either sell for ` 65
(say) i.e. St,u = ` 65 or for ` 40 (say) i.e. St,d = ` 40.

Based on this data, we compute the value of u and d as follows:
S = (1+u)S, or 65 = (1+u)50; Therefore, u = 0.30
Similarly,
S = (1+d)S; or 40 = (1+d)50; Therefore, d = (–) 0.20

LOVELY PROFESSIONAL UNIVERSITY 87