Page 93 - DCOM510_FINANCIAL_DERIVATIVES

Financial Derivatives

Notes Here u has to be greater than the riskless rate of return available in the market (denoted by ‘r’ )
to induce the investor to take risk and invest. However, in case u and d are both greater than r,
the investors would have an opportunity to arbitrage. The investors would borrow heavily and
invest in the stocks. As we have assumed a perfect market, no arbitrage opportunities could
exist. As such, it is imperative that: u > r > d
Similarly, we cannot have a situation where the risk less rate of return is greater than the returns
on risky securities, i.e. we cannot have: r > u > d

The reason is obvious. Under no circumstances, an investor would invest in a riskier security if
he is getting higher return in a risk less security.
Now coming back to our model, if the price of the underlying stock rises, the price of the call
option (with exercise price k) would be:
Ct,u = Max (0, St,u – k)
In the event of the stock price declining,

Ct,d = Max (0, St,d – k)
In other words,
Ct,u = Max [0, (1+u)St–1 – k ]; and
Ct,d = Max [ 0, (1+d)St–1 – k ]

Now, let us build a portfolio comprising equity and debt, which would exactly replicate the
payoff to the call option over a unit period, i.e. exactly equate the value of the call option.
Consider a portfolio comprising purchase of Z number of shares of the optioned stock which is
financed by borrowing ‘M’ at time t-1 at a risk less rate of interest of ‘r’. Algebraically, this
portfolio would be represented as ‘Z S – M’.

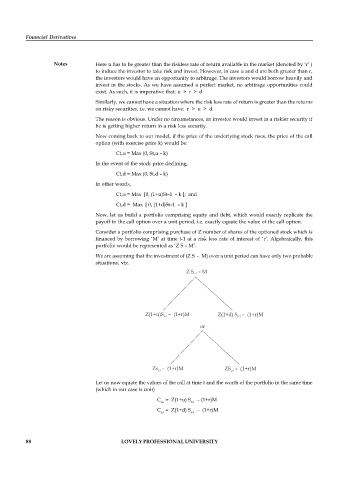
We are assuming that the investment of (Z S – M) over a unit period can have only two probable
situations, viz.
Z S – M
t–1

Z(1+u)S – (1+r)M Z(1+d) S – (1+r)M
t–1 t–1
or

Zs – (1+r)M ZS – (1+r)M
t,1 t,d
Let us now equate the values of the call at time t and the worth of the portfolio in the same time
(which in our case is unit)
C = Z(1+u) S – (1+r)M
t,u t–1
C = Z(1+d) S – (1+r)M
t,d t–1

88 LOVELY PROFESSIONAL UNIVERSITY