Page 95 - DCOM510_FINANCIAL_DERIVATIVES

Financial Derivatives

Notes 6.3.3 Binomial Option Pricing Model (BOPM) for PUTS

The derivation of a unit period BOPM for put options is analogical to that for the call options.
Using the same assumptions and notations as those for calls, we consider the following pricing
process for the put option’s underlying stock:
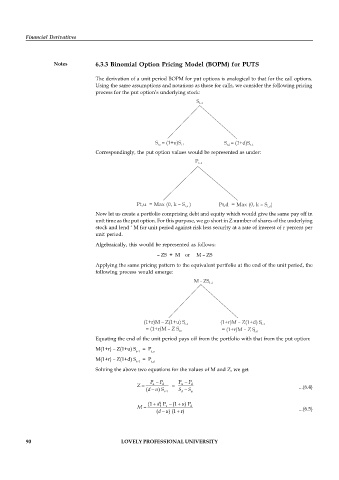
S t–1

S = (1+u)S t–1 S = (1+d)S t–1
t,u t,d
Correspondingly, the put option values would be represented as under:
P t–1

Pt,u = Max (0, k – S ) Pt,d = Max (0, k – S )
t,u t,d
Now let us create a portfolio comprising debt and equity which would give the same pay off in
unit time as the put option. For this purpose, we go short in Z number of shares of the underlying
stock and lend ‘ M for unit period against risk less security at a rate of interest of r percent per
unit period.
Algebraically, this would be represented as follows:
– ZS + M or M – ZS

Applying the same pricing pattern to the equivalent portfolio at the end of the unit period, the
following process would emerge:

M - ZS t–1

(1+r)M – Z(1+u) S t–1 (1+r)M – Z(1+d) S t–1
= (1+r)M – Z S t,u = (1+r)M – Z S t,d
Equating the end of the unit period pays off from the portfolio with that from the put option:
M(1+r) – Z(1+u) S = P
t–1 t,u
M(1+r) – Z(1+d) S = P
t–1 t,d
Solving the above two equations for the values of M and Z, we get

P  P P  P
Z  u d  u d ...(6.4)
u
(d  ) S r-1 S d  S u
(1  ) P  (1  ) P
u
d
M  u d ...(6.5)
(d  ) (1 r)
u

90 LOVELY PROFESSIONAL UNIVERSITY