Page 96 - DCOM510_FINANCIAL_DERIVATIVES

Unit 6: Valuation and Pricing of Options

Thus, we get the debt and equity components of the portfolio that exactly replicates the put over Notes
a time t. The price of the put at time t–1, i.e. at the beginning of the unit period must equal the
algebraic net of the portfolio:
P = M – ZS
Substituting the values of M and Z in the above equation, we get

XP  (1-X) P
P  ...(6.6)
(1  )
r
where

r d u-r

X  and (1-X)
u d u-d

More generally, we may rewrite the formula as under
XP  (1-X) P
P  ...(6.7)
r
(1  )
Illustration: Let us consider the valuation of a call one period prior to expiration. A stock is
currently selling at ` 50 and that after one period it would be selling either for ` 40 or ` 60. The
rate of interest, both for borrowing and lending, is assumed to be 25% for the one period.
Determine the value of the call with an exercise price of ` 50.
Solution: Consider a portfolio consisting of (i) writing two calls; (ii) buying one share of the
stock; and (iii) borrowing ` 32.
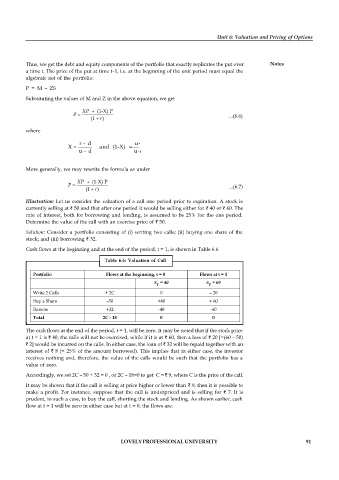
Cash flows at the beginning and at the end of the period, t = 1, is shown in Table 6.6.

Table 6.6: Valuation of Call

Portfolio Flows at the beginning, t = 0 Flows at t = 1
S = 40 S = 60
1 1
Write 2 Calls + 2C 0 – 20
Buy a Share –50 +40 + 60
Borrow +32 -40 -40
Total 2C - 18 0 0

The cash flows at the end of the period, t = 1, will be zero. It may be noted that if the stock price
at t = 1 is ` 40, the calls will not be exercised, while if it is at ` 60, then a loss of ` 20 [=(60 – 50)
` 2] would be incurred on the calls. In either case, the loan of ` 32 will be repaid together with an
interest of ` 8 (= 25% of the amount borrowed). This implies that in either case, the investor
receives nothing and, therefore, the value of the calls would be such that the portfolio has a
value of zero.
Accordingly, we set 2C – 50 + 32 = 0 , or 2C – 18=0 to get C = ` 9, where C is the price of the call.
It may be shown that if the call is selling at price higher or lower than ` 9, then it is possible to
make a profit. For instance, suppose that the call is underpriced and is selling for ` 7. It is
prudent, in such a case, to buy the call, shorting the stock and lending. As shown earlier, cash
flow at t = 1 will be zero in either case but at t = 0, the flows are:

LOVELY PROFESSIONAL UNIVERSITY 91