Page 220 - DCAP108_DIGITAL_CIRCUITS_AND_LOGIC_DESIGNS

Unit 12: A/D and D/A Converters

Applying Millman’s theorem to Figure 12.5, we obtain Notes
4
(
V / R + V /( R / 2) + VR /)
V = 0 0 1 0 2 0
A 1/ R + 0 1/( R / 2) + 1/( R / 4)
0
0
7/R 7
= 0 = = + 1V
1/R + 2/R + 4/R 0 7
0
0
Drawing the equivalent circuits for the other 7-input combinations and applying Millman’s
theorem will lead to the table of voltages shown in Figure 12.3.
12.2:
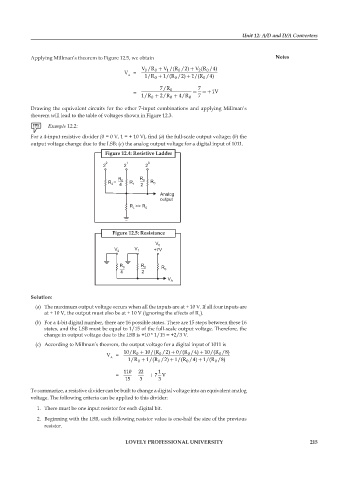
For a 4-input resistive divider (0 = 0 V, 1 = + 10 V), find (a) the full-scale output voltage; (b) the
output voltage change due to the LSB; (c) the analog output voltage for a digital input of 1011.
Figure 12.4: Resistive Ladder

Figure 12.5: Resistance

Solution:
(a) The maximum output voltage occurs when all the inputs are at + 10 V. If all four inputs are
at + 10 V, the output must also be at + 10 V (ignoring the effects of R ).
L
(b) For a 4-bit digital number, there are 16 possible states. There are 15 steps between these 16
states, and the LSB must be equal to 1/15 of the full-scale output voltage. Therefore, the
change in output voltage due to the LSB is +10 * 1/15 = +2/3 V.
(c) According to Millman’s theorem, the output voltage for a digital input of 1011 is
V = 10/R + 0 10/(R 0 / 2) + 0/(R 0 / 4) + 10/(R 0 / 8)
A
8
1/R + 0 1/(R 0 / 2) + 1/(R 0 / 4) + 1//(R 0 /)
110 22 1
= = = + 7 V
15 3 3
To summarize, a resistive divider can be built to change a digital voltage into an equivalent analog
voltage. The following criteria can be applied to this divider:

1. There must be one input resistor for each digital bit.
2. Beginning with the LSB, each following resistor value is one-half the size of the previous
resistor.

LOVELY PROFESSIONAL UNIVERSITY 215