Page 138 - DMTH201_Basic Mathematics-1

Unit 5: Equations of Straight Lines

Notes

Notes

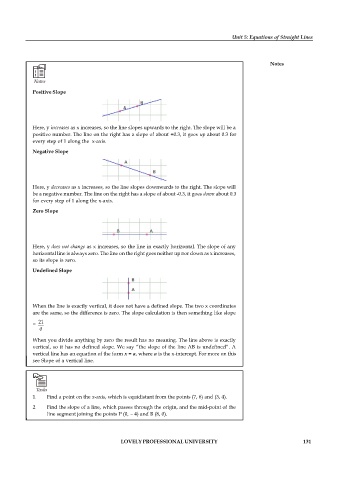
Positive Slope

Here, y increases as x increases, so the line slopes upwards to the right. The slope will be a
positive number. The line on the right has a slope of about +0.3, it goes up about 0.3 for
every step of 1 along the x-axis.

Negative Slope

Here, y decreases as x increases, so the line slopes downwards to the right. The slope will
be a negative number. The line on the right has a slope of about -0.3, it goes down about 0.3
for every step of 1 along the x-axis.
Zero Slope

Here, y does not change as x increases, so the line in exactly horizontal. The slope of any
horizontal line is always zero. The line on the right goes neither up nor down as x increases,
so its slope is zero.
Undefined Slope

When the line is exactly vertical, it does not have a defined slope. The two x coordinates
are the same, so the difference is zero. The slope calculation is then something like slope
21
=
0
When you divide anything by zero the result has no meaning. The line above is exactly
vertical, so it has no defined slope. We say “the slope of the line AB is undefined”. A
vertical line has an equation of the form x = a, where a is the x-intercept. For more on this
see Slope of a vertical line.

Tasks
1. Find a point on the x-axis, which is equidistant from the points (7, 6) and (3, 4).

2. Find the slope of a line, which passes through the origin, and the mid-point of the
line segment joining the points P (0, – 4) and B (8, 0).

LOVELY PROFESSIONAL UNIVERSITY 131