Page 135 - DMTH201_Basic Mathematics-1
P. 135
Basic Mathematics – I
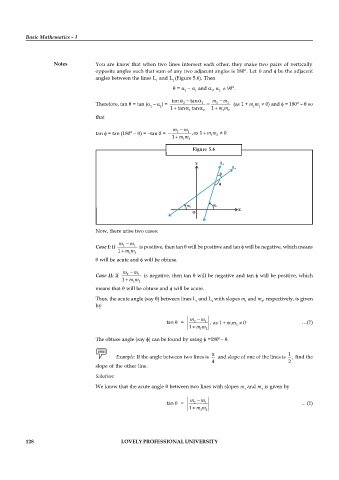
Notes You are know that when two lines intersect each other, they make two pairs of vertically
opposite angles such that sum of any two adjacent angles is 180°. Let and be the adjacent
angles between the lines L and L (Figure 5.6). Then
1 2
= and , 90°.
2 1 1 2
tan tan m m
Therefore, tan = tan ( – ) = 2 1 2 1 (as 1 + m m 0) and = 180° – so
2 1 1 2
1 tan tan 1 m m
1 2 1 2
that
m 2 m 1
tan = tan (180° ) = tan = ,as 1 m m 2 0.
1
1 m m 2
1
Figure 5.6
Now, there arise two cases:
m m
Case I: If 2 1 is positive, then tan will be positive and tan will be negative, which means
1 m m 2
1
will be acute and will be obtuse.
m m
Case II: If 2 1 is negative, then tan will be negative and tan will be positive, which
1 m m
1 2
means that will be obtuse and will be acute.
Thus, the acute angle (say ) between lines L and L with slopes m and m , respectively, is given
1 2 1 2
by
m m
tan = 2 1 , as 1 m m 2 0 …(1)
1
1 m m 2
1
The obtuse angle (say ) can be found by using =180 0 .
1
Example: If the angle between two lines is and slope of one of the lines is , find the
4 2
slope of the other line.
Solution:
We know that the acute angle between two lines with slopes m and m is given by
1 2
m m
tan = 2 1 ... (1)
1 m m 2
1
128 LOVELY PROFESSIONAL UNIVERSITY