Page 139 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes 5.3 Various Forms of the Equation of a Line

You know that every line in a plane contains infinite poinjts on it.
The general equation of a line can be reduced into various forms of the equation of a line. In all
forms, slope is represented by m, the x-intercept by a, and the y-intercept by b. The Following are
the different forms of the equation of a line.
Slope-intercept form

Intercept form
Normal form

Notes The standard form coefficients A, B, and C have no particular graphical significance.
As we all know that you can find the equation of the line If two points on the line are given
and If one point on the line and the slope is given.

5.3.1 Horizontal and Vertical Lines

The general equation of straight line is given by: Ax + By = C
a - If we set A to zero in the general equation, we obtain an equation in y only of the form
By = C

which gives y = C/B = k; k is a constant. This is a horizontal line with slope 0 and passes through
all points with y coordinate equal to k.
b - If we set B to zero in the general equation, we obtain

Ax = C
which gives x = C/A = h; h is constant. This is a vertical line with undefined slope and passes
through all points with x coordinate equal to h

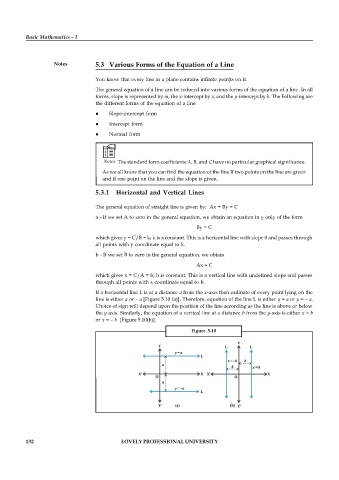
If a horizontal line L is at a distance a from the x-axis then ordinate of every point lying on the
line is either a or a [Figure 5.10 (a)]. Therefore, equation of the line L is either y = a or y = a.
Choice of sign will depend upon the position of the line according as the line is above or below
the y-axis. Similarly, the equation of a vertical line at a distance b from the y-axis is either x = b
or x = b [Figure 5.10(b)].
Figure 5.10

132 LOVELY PROFESSIONAL UNIVERSITY