Page 139 - DMTH202_BASIC_MATHEMATICS_II
P. 139
Basic Mathematics-II
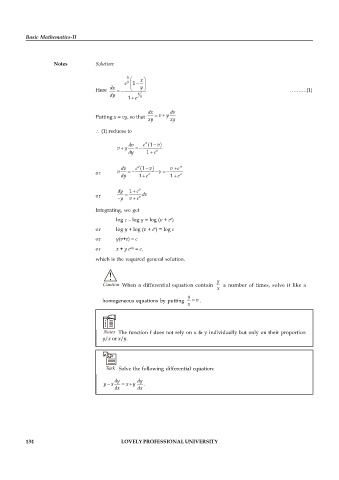
Notes Solution:
x
e y 1 x
dx y
Here ……….(1)
dy x y
1 e
dx dv
v
Putting x = vy, so that y
xy xy
(1) reduces to
dv e v 1 v
v y v
dy 1 e
dv e v 1 v v e v
v
or v v v
dy 1 e 1 e
dy 1 e v
or v dv
y v e
Integrating, we get
v
log c – log y = log (v + e )
or log y + log (v + e ) = log c
v
or y(v+e) = c
or x + y e = c.
e/y
which is the required general solution.
!
y
Caution When a differential equation contain a number of times, solve it like a
x
y
homogeneous equations by putting v .
x
Notes The function f does not rely on x & y individually but only on their proportion
y/x or x/y.
Task Solve the following differential equation:
dy dy
y x y .
x
dx dx
134 LOVELY PROFESSIONAL UNIVERSITY