Page 152 - DMTH202_BASIC_MATHEMATICS_II

Unit 11: Linear Differential Equations of First Order

Notes
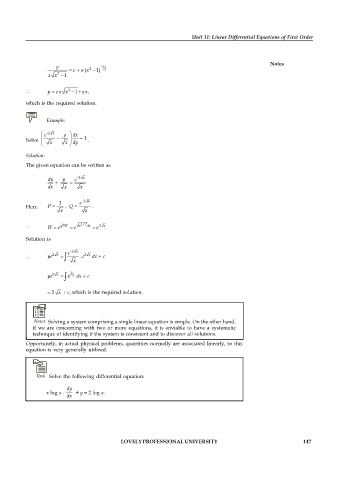
y 2 1
a
c
  (x 1) 2
x x 2  1
 y  c x x 2  ax ,
1
which is the required solution.

Example:

 e  2 x y  dx
    1
Solve  x x  dy .
 
Solution:
The given equation can be written as

dy y e  2 x
 
dx x x

1 e  2 x
Here P  , Q  .
x x

/
 IF  e Pdf  e x 1 2 dx  e 2 x .
Solution is

e  2 x 2 x
2 x
 ye   x .e dx  c

1
ye 2 x   x 2 dx  c

 2 x  , c which is the required solution.

Notes Solving a system comprising a single linear equation is simple. On the other hand,
if we are concerning with two or more equations, it is enviable to have a systematic
technique of identifying if the system is consistent and to discover all solutions.
Opportunely, in actual physical problems, quantities normally are associated linearly, so this
equation is very generally utilized.

Task Solve the following differential equation:

dy
x log x . + y = 2 log x.
dx

LOVELY PROFESSIONAL UNIVERSITY 147