Page 107 - DMTH201_Basic Mathematics-1
P. 107
Basic Mathematics – I
Notes
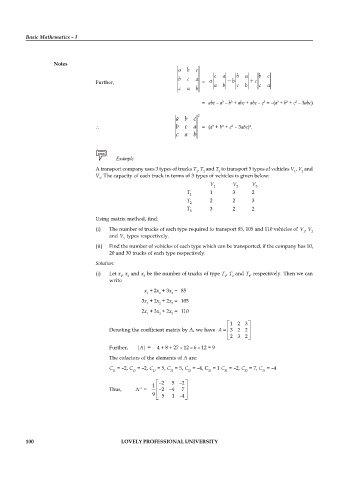
a b c
c a b a b c
b c a a b c
Further, = a b c b c a
c a b
3
3
3
3
= abc – a – b + abc + abc – c = –(a + b + c – 3abc)
2
3
2
a b c
b c a = (a + b + c – 3abc) .
3
2
3
3
c a b
Example
A transport company uses 3 types of trucks T , T and T to transport 3 types of vehicles V , V and
1 2 3 1 2
V . The capacity of each truck in terms of 3 types of vehicles is given below:
3
V V V
1 2 3
T 1 3 2
1
T 2 2 3
2
T 3 2 2
3
Using matrix method, find:
(i) The number of trucks of each type required to transport 85, 105 and 110 vehicles of V , V
1 2
and V types respectively.
3
(ii) Find the number of vehicles of each type which can be transported, if the company has 10,
20 and 30 trucks of each type respectively.
Solution:
(i) Let x , x and x be the number of trucks of type T , T and T , respectively. Then we can
1 2 3 1 2 3
write
x + 2x + 3x = 85
1 2 3
3x + 2x + 2x = 105
1 2 3
2x + 3x + 2x = 110
1 2 3
1 2 3
Denoting the coefficient matrix by A, we have A 3 2 2
2 3 2
Further, |A| = 4 + 8 + 27 – 12 – 6 – 12 = 9
The cofactors of the elements of A are:
C = –2, C = –2, C = 5, C = 5, C = –4, C = 1 C = –2, C = 7, C = –4
11 12 13 21 22 23 31 32 33
2 5 2
1
Thus, A = 2 4 7
–1
9 5 1 4
100 LOVELY PROFESSIONAL UNIVERSITY