Page 153 - DMTH201_Basic Mathematics-1
P. 153
Basic Mathematics – I
Notes Solving (1) and (2), we get x = 1 and y = 4 so that point of intersection of the two lines is Q (1, 4).
Now, distance of line (1) from the point P (4, 1) along the line (2)
= the distance between the points P (4, 1) and Q (1, 4).
= (1 4) 2 (4 1) 2 3 2 units.
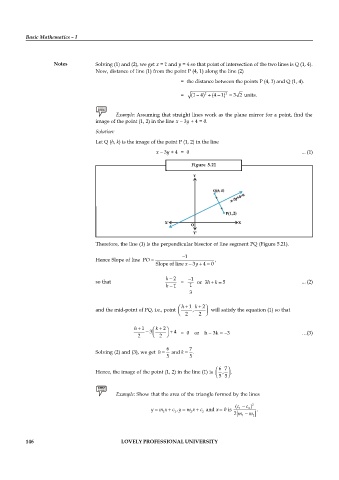
Example: Assuming that straight lines work as the plane mirror for a point, find the
image of the point (1, 2) in the line x 3y + 4 = 0.
Solution:
Let Q (h, k) is the image of the point P (1, 2) in the line
x 3y + 4 = 0 ... (1)
Figure 5.21
Therefore, the line (1) is the perpendicular bisector of line segment PQ (Figure 5.21).
1
Hence Slope of line PO ,
Slope of line x 3y 4 0
k 2 1
so that = or 3h k 5 ... (2)
h 1 1
3
h 1 k 2
and the mid-point of PQ, i.e., point , will satisfy the equation (1) so that
2 2
h 1 k 2
3 4 = 0 or h 3k = 3 …(3)
2 2
6 7
Solving (2) and (3), we get h and k .
5 5
6 7
Hence, the image of the point (1, 2) in the line (1) is , .
5 5
Example: Show that the area of the triangle formed by the lines
(c c ) 2
y m x c ,y m x c and x 0 is 1 2 .
1 1 2 2
2 m 1 m 2
146 LOVELY PROFESSIONAL UNIVERSITY