Page 154 - DMTH201_Basic Mathematics-1
P. 154
Unit 5: Equations of Straight Lines
Solution: Notes
Given lines are
y = m x + c ... (1)
1 1
y = m x + c ... (2)
2 2
x = 0 ... (3)
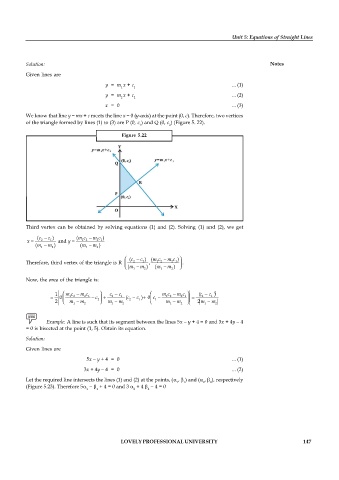
We know that line y = mx + c meets the line x = 0 (y-axis) at the point (0, c). Therefore, two vertices
of the triangle formed by lines (1) to (3) are P (0, c ) and Q (0, c ) (Figure 5. 22).
1 2
Figure 5.22
Third vertex can be obtained by solving equations (1) and (2). Solving (1) and (2), we get
(c c ) (m c m c )
x 2 1 and y 1 2 2 1
(m 1 m 2 ) (m 1 m 2 )
(c c ) (m c m c )
Therefore, third vertex of the triangle is R 2 1 , 1 2 2 1 .
(m m ) (m m )
1 2 1 2
Now, the area of the triangle is:
1 m c m c c c m c m c ( c c ) 2
0 1 2 2 1 c 2 1 (c c ) 0 c 1 2 2 1 2 1
2 m m 2 m m 2 1 1 m m 2 m m
1 2 1 2 1 2 1 2
Example: A line is such that its segment between the lines 5x y + 4 = 0 and 3x + 4y 4
= 0 is bisected at the point (1, 5). Obtain its equation.
Solution:
Given lines are
5x y + 4 = 0 ... (1)
3x + 4y 4 = 0 ... (2)
Let the required line intersects the lines (1) and (2) at the points, ( , ) and ( , ), respectively
1 1 2 2
(Figure 5.23). Therefore 5 + 4 = 0 and 3 + 4 4 = 0
1 1 2 2
LOVELY PROFESSIONAL UNIVERSITY 147