Page 217 - DMTH201_Basic Mathematics-1
P. 217
Basic Mathematics – I
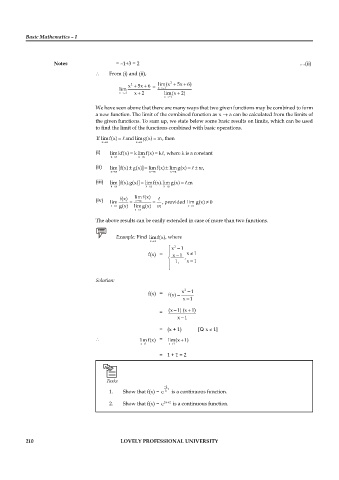
Notes = –1+3 = 2 ....(ii)
From (i) and (ii),
=
We have seen above that there are many ways that two given functions may be combined to form
a new function. The limit of the combined function as x a can be calculated from the limits of
the given functions. To sum up, we state below some basic results on limits, which can be used
to find the limit of the functions combined with basic operations.
(i)
(ii)
(iii)
(iv)
The above results can be easily extended in case of more than two functions.
Example: Find , where
f(x) =
Solution:
f(x) =
=
= (x + 1) [Q x 1]
=
= 1 + 1 = 2
1. Show that f(x) = is a continuous function.
2. Show that f(x) = is a continuous function.
210 LOVELY PROFESSIONAL UNIVERSITY