Page 234 - DMTH201_Basic Mathematics-1

Unit 8: Continuity

8.2.1 Continuous Functions Notes

A function is continuous if it has no breaks. On this page we’ll first look at some common
continuous functions, and then show you the discontinuous ones that you’re likely to come
across in high school mathematics.

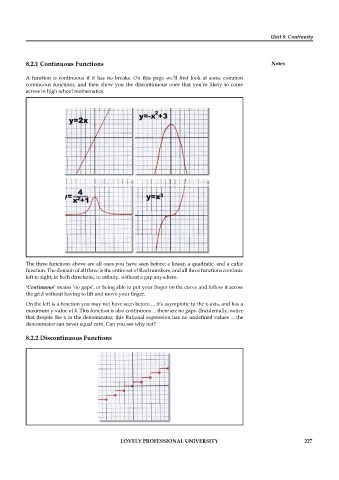
The three functions above are all ones you have seen before: a linear, a quadratic, and a cubic
function. The domain of all three is the entire set of Real numbers, and all three functions continue
left to right, in both directions, to infinity, without a gap anywhere.

‘Continuous’ means ‘no gaps’, or being able to put your finger on the curve and follow it across

the grid without having to lift and move your finger.
On the left is a function you may not have seen before ... it’s asymptotic to the x-axis, and has a
maximum y-value of 4. This function is also continuous ... there are no gaps. (Incidentally, notice
that despite the x in the denominator, this Rational expression has no undefined values ... the

denominator can never equal zero. Can you see why not?

8.2.2 Discontinuous Functions

LOVELY PROFESSIONAL UNIVERSITY 227