Page 121 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

Notes 9.4 Differential Equations of the First Order and First Degree

All differential equations of the first order and first degree cannot be solved; they can be solved,
however, if they belong to one or the other of the following standard forms (categories) by the
standard methods:
1. Equations in which the variables are separable,
2. Homogeneous equations,

3. Linear Equations,
4. Exact Equations.

9.4.1 Geometric Meaning of a Differential Equation of the First Order
and First Degree

 dy 
f x,y,
Let    0 ………..(1)
 dx 
be a differential equation of the first order and first degree since the direction of a curve at a
particular point is determined by drawing a tangent line at that point, i.e., its slope is given
dy
by at that particular point.
dx
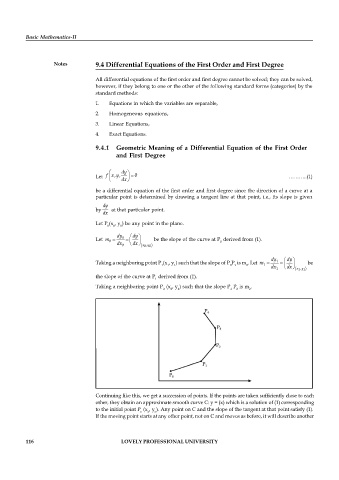
Let P (x , y ) be any point in the plane.
0 0 0
dy 0  dy 
Let m 0     be the slope of the curve at P derived from (1).
dx 0  dx   x ,y  0 0
0
dy  dy 
Taking a neighboring point P (x , y ) such that the slope of P P is m . Let m 1  1    be
1 1 1 0 1 0
dx 1  dx   x ,y  1
1
the slope of the curve at P derived from (1).
1
Taking a neighboring point P (x , y ) such that the slope P P is m .
2 2 2 1 2 2

Continuing like this, we get a succession of points. If the points are taken sufficiently close to each
other, they obtain an approximate smooth curve C: y = (x) which is a solution of (1) corresponding
to the initial point P (x , y ). Any point on C and the slope of the tangent at that point satisfy (1).
o o o
If the moving point starts at any other point, not on C and moves as before, it will describe another

116 LOVELY PROFESSIONAL UNIVERSITY