Page 43 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

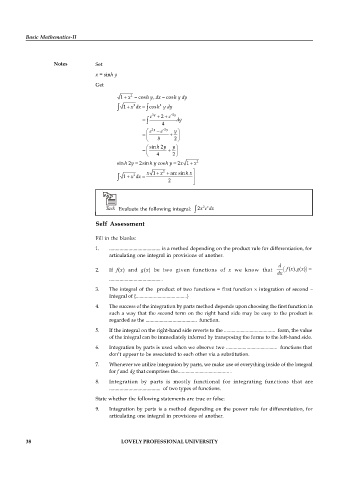
Notes Set
x = sinh y
Get

2
,

1 x  cosh y dx  cosh y dy
2
2
 1 x dx   cosh y dy

e 2y  2  e  2y
  dy
4
 e 2y  e  2y y 
   
 8 2 
h
 sin 2y y 
   
 4 2 
h

sin 2y  2sinh y cosh y  2x 1 x 2
2
x 1 x  arc sinh x 

2
 1 x dx  2   

2 x

Task Evaluate the following integral: 2x e dx
Self Assessment
Fill in the blanks:
1. ........................................ is a method depending on the product rule for differentiation, for
articulating one integral in provisions of another.
d
2. If f(x) and g(x) be two given functions of x we know that  ( ). ( )f x g x  
dx
........................................ .
3. The integral of the product of two functions = first function  integration of second –
Integral of {........................................}

4. The success of the integration by parts method depends upon choosing the first function in
such a way that the second term on the right hand side may be easy to the product is
regarded as the ........................................ function.
5. If the integral on the right-hand side reverts to the ........................................ form, the value
of the integral can be immediately inferred by transposing the forms to the left-hand side.
6. Integration by parts is used when we observe two ........................................ functions that
don’t appear to be associated to each other via a substitution.

7. Whenever we utilize integration by parts, we make use of everything inside of the integral
for f and dg that comprises the........................................ .
8. Integration by parts is mostly functional for integrating functions that are
........................................ of two types of functions.
State whether the following statements are true or false:
9. Integration by parts is a method depending on the power rule for differentiation, for
articulating one integral in provisions of another.

38 LOVELY PROFESSIONAL UNIVERSITY