Page 174 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

Notes This unit deals only with linear association between the two variables X and Y. We shall measure
the degree of linear association by the Karl Pearson’s formula for the coefficient of linear
correlation.

9.1.2 Karl Pearson’s Coefficient of Linear Correlation

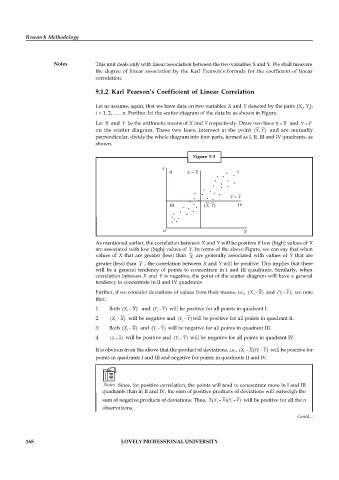
Let us assume, again, that we have data on two variables X and Y denoted by the pairs (X , Y ),
i i
i = 1, 2, ...... n. Further, let the scatter diagram of the data be as shown in Figure.
Let X and Y be the arithmetic means of X and Y respectively. Draw two lines X  X and Y  Y
on the scatter diagram. These two lines, intersect at the point ( , )X Y and are mutually
perpendicular, divide the whole diagram into four parts, termed as I, II, III and IV quadrants, as
shown.

Figure 9.3

Y
II X = X I

Y = Y
Y
X,
III ( ) IV

O X

As mentioned earlier, the correlation between X and Y will be positive if low (high) values of X
are associated with low (high) values of Y. In terms of the above Figure, we can say that when
values of X that are greater (less) than X are generally associated with values of Y that are
greater (less) than Y , the correlation between X and Y will be positive. This implies that there
will be a general tendency of points to concentrate in I and III quadrants. Similarly, when
correlation between X and Y is negative, the point of the scatter diagram will have a general
tendency to concentrate in II and IV quadrants.

X
Further, if we consider deviations of values from their means, i.e., (X – ) and (Y – ), we note
Y
i i
that:
Y
X
1. Both (X – ) and (Y – ) will be positive for all points in quadrant I.
i i
2. (X – ) will be negative and (Y – ) will be positive for all points in quadrant II.
Y
X
i i
3. Both (X – ) and (Y – ) will be negative for all points in quadrant III.
X
Y
i i
4. (X – ) will be positive and (Y – ) will be negative for all points in quadrant IV.
Y
X
i i
X
It is obvious from the above that the product of deviations, i.e., (X – )(Y – ) will be positive for
Y
i i
points in quadrants I and III and negative for points in quadrants II and IV.
Notes Since, for positive correlation, the points will tend to concentrate more in I and III
quadrants than in II and IV, the sum of positive products of deviations will outweigh the
sum of negative products of deviations. Thus, (X – )(Y – ) will be positive for all the n
Y
X
i i
observations.
Contd...
168 LOVELY PROFESSIONAL UNIVERSITY