Page 176 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

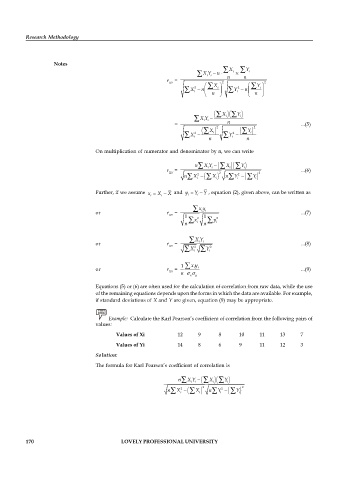
Notes å
å X Y - × X i å Y i
´
n
i
i
r = n n
XY æ å X ö 2 æ å Y ö 2
å X - n ç i ÷ å Y - n ç i ÷
2
2
i
è n ø i è n ø
(å X )(å Y )
å X Y - i i
i
i
= (å ) 2 n (å ) 2 ...(5)
å X - X i å Y - Y i
2
2
i
n i n
On multiplication of numerator and denominator by n, we can write
nå X Y - (å X )(å Y )
r = i i i i ...(6)
nå X - (å X ) nå Y - (å Y )
XY 2 2
2
2
i i i i
Further, if we assume x  X - X and y  Y - Y , equation (2), given above, can be written as
i i i i
å x y
or r = i i ...(7)
XY 1 å 2 1 å 2
n x i n y i
å X Y
or r = i i ...(8)
å X i å Y i 2
XY
2
1  x y
or r = i i ...(9)
XY
n  
x y
Equations (5) or (6) are often used for the calculation of correlation from raw data, while the use
of the remaining equations depends upon the forms in which the data are available. For example,
if standard deviations of X and Y are given, equation (9) may be appropriate.

Example: Calculate the Karl Pearson’s coefficient of correlation from the following pairs of
values:
Values of Xi 12 9 8 10 11 13 7
Values of Yi 14 8 6 9 11 12 3
Solution:

The formula for Karl Pearson’s coefficient of correlation is
nå X Y - (å X i )(å Y i )
i
i
nå X - (å X ) 2 nå Y - (å Y ) 2
2
2
i i i i

170 LOVELY PROFESSIONAL UNIVERSITY