Page 179 - DMGT404 RESEARCH_METHODOLOGY

Unit 9: Correlation and Regression

Notes
-
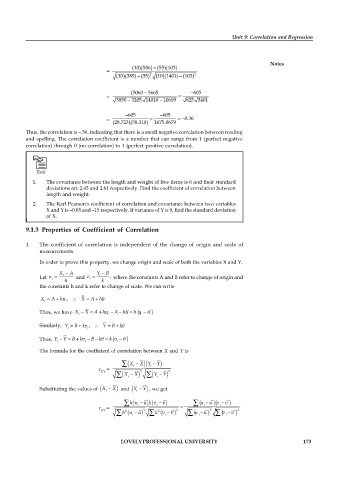
(10)(506) (55)(103)
=
-
-
(10)(385) (55) 2 (10)(1401) (103) 2
-
(5060 5665 - 605
= 
-
-
3850 3205 14010 10609 825 3401
- 605 - 605
=   - 0.36
(28.723)(58.318) 1675.0679
Thus, the correlation is –.36, indicating that there is a small negative correlation between reading
and spelling. The correlation coefficient is a number that can range from 1 (perfect negative
correlation) through 0 (no correlation) to 1 (perfect positive correlation).

Task

1. The covariance between the length and weight of five items is 6 and their standard
deviations are 2.45 and 2.61 respectively. Find the coefficient of correlation between
length and weight.
2. The Karl Pearson's coefficient of correlation and covariance between two variables
X and Y is –0.85 and –15 respectively. If variance of Y is 9, find the standard deviation
of X.

9.1.3 Properties of Coefficient of Correlation

1. The coefficient of correlation is independent of the change of origin and scale of
measurements.
In order to prove this property, we change origin and scale of both the variables X and Y.
X - A Y - B
Let u  i and v  i , where the constants A and B refer to change of origin and
i
i
h k
the constants h and k refer to change of scale. We can write
X  A hu i , \ X  A hu
+
+
i
Thus, we have X - X  A hu - A hu  ( h u - ) u
-
+
i
i
i
Similarly, Y  B kv , \ Y  B kv
+
+
i i
+
Thus, Y - Y  B kv - B kv  k v - ) v
-
i i ( i
The formula for the coefficient of correlation between X and Y is
å (X - X Y - Y )
)( i
i
r =
å (X - X ) å ( i Y )
XY 2 2
Y -
i
Substituting the values of (X - X ) and ( i Y ) , we get
Y -
i
å h u - u k v - ) v å ( i u v - ) v
u -
)( i
( i
) ( i
r = 
XY å h u - ) u 2 å k v - ) v 2 å ( i u - ) u 2 å ( i v - ) v 2
2
2
( i
( i
LOVELY PROFESSIONAL UNIVERSITY 173