Page 234 - DECO401_MICROECONOMIC_THEORY_ENGLISH

Unit-11: Concepts of Revenue

Notes
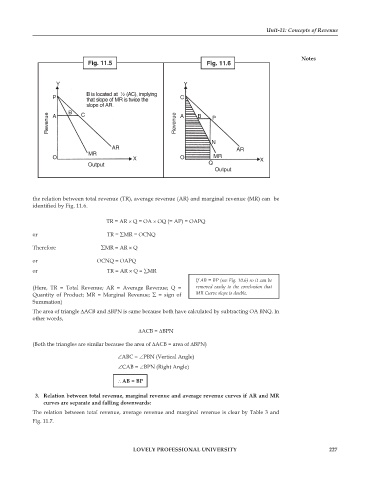
Fig. 11.5 Fig. 11.6

Y Y
B is located at ½ (AC), implying
P that slope of MR is twice the C
slope of AR.
B C A B
Revenue Revenue P
A

N
AR AR
MR
O X O MR X
Output Q
Output

the relation between total revenue (TR), average revenue (AR) and marginal revenue (MR) can be
identified by Fig. 11.6.

TR = AR × Q = OA × OQ (= AP) = OAPQ

or TR = ∑MR = OCNQ
Therefore ∑MR = AR × Q

or OCNQ = OAPQ
or TR = AR × Q = ∑MR
If AB = BP (see Fig. 10.6) so it can be
(Here, TR = Total Revenue; AR = Average Revenue; Q = removed easily to the conclusion that
Quantity of Product; MR = Marginal Revenue; ∑ = sign of MR Curve slope is double.
Summation)
The area of triangle ∆ACB and ∆BPN is same because both have calculated by subtracting OA BNQ. In
other words,

∆ACB = ∆BPN
(Both the triangles are similar because the area of ∆ACB = area of ∆BPN)

∠ABC = ∠PBN (Vertical Angle)
∠ CAB = ∠BPN (Right Angle)

∴AB = BP

3. Relation between total revenue, marginal revenue and average revenue curves if AR and MR
curves are separate and falling downwards:
The relation between total revenue, average revenue and marginal revenue is clear by Table 3 and
Fig. 11.7.

LOVELY PROFESSIONAL UNIVERSITY 227