Page 282 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes There are four kinds of changes, or variations, involved in time series analysis. They are:
(i) Secular trend
(ii) Cyclical fluctuation (variation)
(iii) Seasonal variation
(iv) Irregular variation
With the secular trend, the value of the variable tends to increase or decrease over a long period of
time. The steady increase in the cost of living recorded by the consumer price index is an example of
secular trend. From year to year, the cost of living varies a great deal; but, if we consider a long-term
period, we see that the trend is towards steady increase. Other examples of secular trend are steady
increase of population over a period of time, steady growth of agricultural food production in India
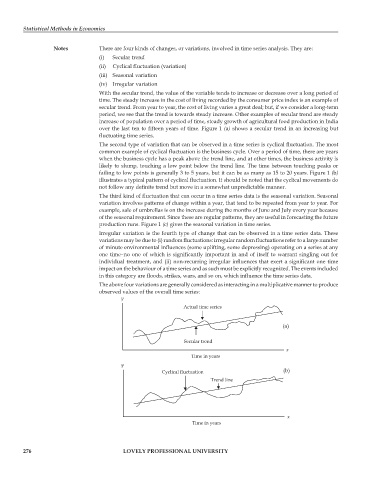
over the last ten to fifteen years of time. Figure 1 (a) shows a secular trend in an increasing but
fluctuating time series.
The second type of variation that can be observed in a time series is cyclical fluctuation. The most
common example of cyclical fluctuation is the business cycle. Over a period of time, there are years
when the business cycle has a peak above the trend line, and at other times, the business activity is
likely to slump, touching a low point below the trend line. The time between touching peaks or
failing to low points is generally 3 to 5 years, but it can be as many as 15 to 20 years. Figure 1 (b)
illustrates a typical pattern of cyclical fluctuation. It should be noted that the cyclical movements do
not follow any definite trend but move in a somewhat unpredictable manner.
The third kind of fluctuation that can occur in a time series data is the seasonal variation. Seasonal
variation involves patterns of change within a year, that tend to be repeated from year to year. For
example, sale of umbrellas is on the increase during the months of June and July every year because
of the seasonal requirement. Since these are regular patterns, they are useful in forecasting the future
production runs. Figure 1 (c) gives the seasonal variation in time series.
Irregular variation is the fourth type of change that can be observed in a time series data. These
variations may be due to (i) random fluctuations: irregular random fluctuations refer to a large number
of minute environmental influences (some uplifting, some depressing) operating on a series at any
one time–no one of which is significantly important in and of itself to warrant singling out for
individual treatment, and (ii) non-recurring irregular influences that exert a significant one time
impact on the behaviour of a time series and as such must be explicitly recognized. The events included
in this category are floods, strikes, wars, and so on, which influence the time series data.
The above four variations are generally considered as interacting in a multiplicative manner to produce
observed values of the overall time series:
y
Actual time series

(a)

Secular trend
x
Time in years
y
Cyclical fluctuation (b)
Trend line

x
Time in years

276 LOVELY PROFESSIONAL UNIVERSITY