Page 283 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 22: Time Series Analysis—Introduction and Components of Time Series

y Notes

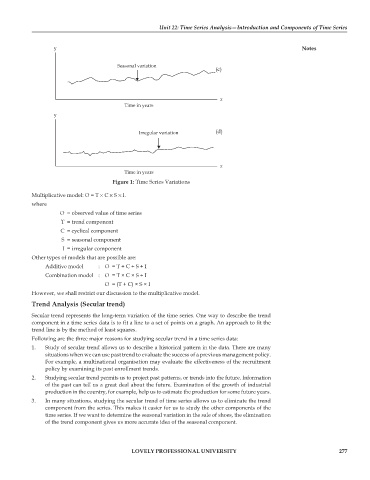
Seasonal variation
(c)

x
Time in years
y

Irregular variation (d)

x
Time in years
Figure 1: Time Series Variations

Multiplicative model: O = T × C × S × I.
where
O = observed value of time series
T = trend component
C = cyclical component
S = seasonal component
I = irregular component
Other types of models that are possible are:
Additive model : O = T + C + S + I
Combination model : O = T × C × S + I
O = (T + C) × S × I
However, we shall restrict our discussion to the multiplicative model.
Trend Analysis (Secular trend)
Secular trend represents the long-term variation of the time series. One way to describe the trend
component in a time series data is to fit a line to a set of points on a graph. An approach to fit the
trend line is by the method of least squares.
Following are the three major reasons for studying secular trend in a time series data:
1. Study of secular trend allows us to describe a historical pattern in the data. There are many
situations when we can use past trend to evaluate the success of a previous management policy.
For example, a multinational organisation may evaluate the effectiveness of the recruitment
policy by examining its past enrollment trends.
2. Studying secular trend permits us to project past patterns, or trends into the future. Information
of the past can tell us a great deal about the future. Examination of the growth of industrial
production in the country, for example, help us to estimate the production for some future years.
3. In many situations, studying the secular trend of time series allows us to eliminate the trend
component from the series. This makes it easier for us to study the other components of the
time series. If we want to determine the seasonal variation in the sale of shoes, the elimination
of the trend component gives us more accurate idea of the seasonal component.

LOVELY PROFESSIONAL UNIVERSITY 277