Page 285 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 22: Time Series Analysis—Introduction and Components of Time Series

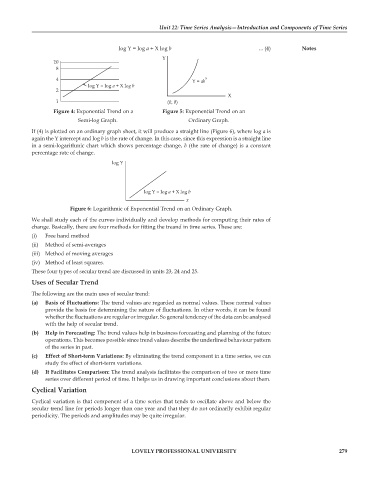
log Y = log a + X log b ... (4) Notes

Y
10
8
4 x
Y= ab
logY=log +Xlog b
a
2
X
1 (0, 0)
Figure 4: Exponential Trend on a Figure 5: Exponential Trend on an
Semi-log Graph. Ordinary Graph.
If (4) is plotted on an ordinary graph sheet, it will produce a straight line (Figure 6), where log a is
again the Y intercept and log b is the rate of change. In this case, since this expression is a straight line
in a semi-logarithmic chart which shows percentage change, b (the rate of change) is a constant
percentage rate of change.
log Y

log Y = log + X log b
a
x
Figure 6: Logarithmic of Exponential Trend on an Ordinary Graph.

We shall study each of the curves individually and develop methods for computing their rates of
change. Basically, there are four methods for fitting the treand in time series. These are:
(i) Free hand method
(ii) Method of semi-averages
(iii) Method of moving averages
(iv) Method of least squares.
These four types of secular trend are discussed in units 23, 24 and 25.
Uses of Secular Trend

The following are the main uses of secular trend:
(a) Basis of Fluctuations: The trend values are regarded as normal values. These normal values
provide the basis for determining the nature of fluctuations. In other words, it can be found
whether the fluctuations are regular or irregular. So general tendency of the data can be analysed
with the help of secular trend.
(b) Help in Forecasting: The trend values help in business forecasting and planning of the future
operations. This becomes possible since trend values describe the underlined behaviour pattern
of the series in past.
(c) Effect of Short-term Variations: By eliminating the trend component in a time series, we can
study the effect of short-term variations.
(d) It Facilitates Comparison: The trend analysis facilitates the comparison of two or more time
series over different period of time. It helps us in drawing important conclusions about them.
Cyclical Variation
Cyclical variation is that component of a time series that tends to oscillate above and below the
secular trend line for periods longer than one year and that they do not ordinarily exhibit regular
periodicity. The periods and amplitudes may be quite irregular.

LOVELY PROFESSIONAL UNIVERSITY 279