Page 290 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 22: Time Series Analysis—Introduction and Components of Time Series

3. Convert these averages into chain relatives on the basis of the first season. Notes
4. Calculate the chain relative of the first season on the basis of the last season.
5. A correction is applied to each of the relatives that have been computed in the earlier step. For
this correction, the chain relative of the first season calculated by the first method is deducted
from the chain relative of the first season calculated by the second method. The difference is
divided by the number of seasons in a year. The resulting figure multiplied by 1, 2, 3 etc. is
deducted respectively from the chain relatives of the 2 , 3 , 4 , etc.
nd
th
rd
6. The seasonal indices are obtained when the corrected chain relatives are expressed as percentage
of their relative averages.
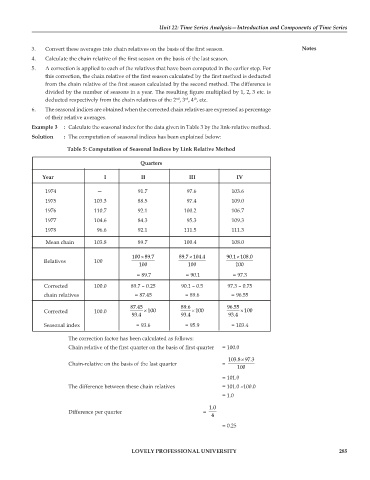
Example 3 : Calculate the seasonal index for the data given in Table 3 by the link-relative method.
Solution : The computation of seasonal indices has been explained below:
Table 5: Computation of Seasonal Indices by Link Relative Method

Quarters

Year I II III IV

1974 — 91.7 97.6 103.6
1975 103.3 88.5 97.4 109.0
1976 110.7 92.1 100.2 106.7
1977 104.6 84.3 95.3 109.3
1978 96.6 92.1 111.5 111.3
Mean chain 103.8 89.7 100.4 108.0

100 × 89.7 89.7 × 104.4 90.1 × 108.0
Relatives 100
100 100 100
= 89.7 = 90.1 = 97.3
Corrected 100.0 89.7 – 0.25 90.1 – 0.5 97.3 – 0.75
chain relatives = 87.45 = 89.6 = 96.55

87.45 89.6 96.55
Corrected 100.0 × 100 × 100 × 100
93.4 93.4 93.4
Seasonal index = 93.6 = 95.9 = 103.4

The correction factor has been calculated as follows:
Chain relative of the first quarter on the basis of first quarter = 100.0

103.8 × 97.3
Chain-relative on the basis of the last quarter =
100
= 101.0
The difference between these chain relatives = 101.0 –100.0
= 1.0
1.0
Difference per quarter =
4
= 0.25

LOVELY PROFESSIONAL UNIVERSITY 285