Page 128 - DMTH401_REAL ANALYSIS

Real Analysis

Notes or equivalently by:
ì  1 if x < 0
ï
f(x) = í 0 if x  0
ï 1 if x > 0
î
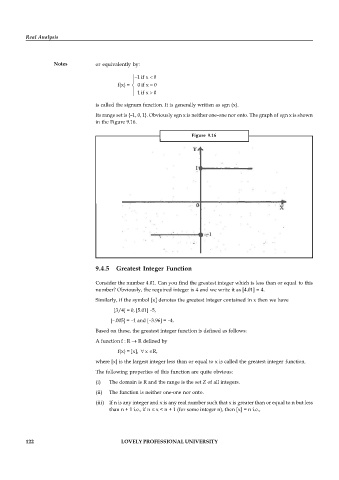
is called the signum function. It is generally written as sgn (x).

Its range set is {–1, 0, 1}. Obviously sgn x is neither one-one nor onto. The graph of sgn x is shown
in the Figure 9.16.

Figure 9.16

9.4.5 Greatest Integer Function

Consider the number 4.01. Can you find the greatest integer which is less than or equal to this
number? Obviously, the required integer is 4 and we write it as [4.01] = 4.
Similarly, if the symbol [x] denotes the greatest integer contained in x then we have

[3/4] = 0, [5.01] –5,
[–.005] = –1 and [–3.96] = –4.
Based on these, the greatest integer function is defined as follows:
A function f : R  R defined by
f(x) = [x], " x R,

where [x] is the largest integer less than or equal to x is called the greatest integer function.
The following properties of this function are quite obvious:
(i) The domain is R and the range is the set Z of all integers.

(ii) The function is neither one-one nor onto.
(iii) If n is any integer and x is any real number such that x is greater than or equal to n but less
than n + 1 i.e., if n  x < n + 1 (for some integer n), then [x] = n i.e.,

122 LOVELY PROFESSIONAL UNIVERSITY