Page 125 - DMTH401_REAL ANALYSIS

Unit 9: Functions

x y
(iii) (a ) = a xY Notes
x
x
x
(iv) a b – (ab) , a > 0, b > 0.
Denote E (I) = e, so that log e = 1. The number e is an irrational number and its approximation say
up to five places of decimals is 2.71828. Thus
x
e = Exp (x log e) = Exp (x).
x
Thus Exp (x) is also denoted as e and we write for each a > 0, a = e log a
x
x
x
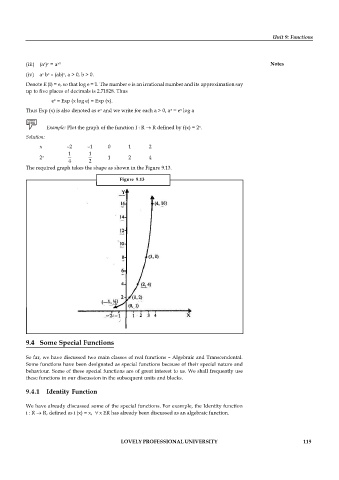
Example: Plot the graph of the function I : R  R defined by f(x) = 2 .
Solution:
x –2 –1 0 1 2
1 1
2 x 1 2 4
4 2
The required graph takes the shape as shown in the Figure 9.13.

Figure 9.13

9.4 Some Special Functions

So far, we have discussed two main classes of real functions – Algebraic and Transcendental.
Some functions have been designated as special functions because of their special nature and
behaviour. Some of these special functions are of great interest to us. We shall frequently use
these functions in our discussion in the subsequent units and blocks.

9.4.1 Identity Function

We have already discussed some of the special functions. For example, the Identity function
i : R  R, defined as i (x) = x, " x ER has already been discussed as an algebraic function.

LOVELY PROFESSIONAL UNIVERSITY 119