Page 126 - DMTH401_REAL ANALYSIS

Real Analysis

Notes However, this function is sometimes, referred to as a special function because of its special
characteristics, which are as follows:
(i) Domain of i = Range of i = Codomain of i

(ii) The function i is one-one and onto. Hence it has an inverse i which is also one-one and
–1
onto.
(iii) The function i is invertible

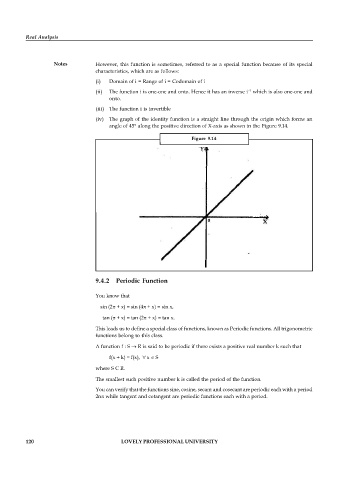
(iv) The graph of the identity function is a straight line through the origin which forms an
angle of 45° along the positive direction of X-axis as shown in the Figure 9.14.

Figure 9.14

9.4.2 Periodic Function

You know that

sin (2 + x) = sin (4 + x) = sin x,
tan ( + x) = tan (2 + x) = tan x.

This leads us to define a special class of functions, known as Periodic functions. All trigonometric
functions belong to this class.

A function f : S  R is said to be periodic if there exists a positive real number k such that
f(x + k) = f(x), " x S
where S C R.

The smallest such positive number k is called the period of the function.
You can verify that the functions sine, cosine, secant and cosecant are periodic each with a period
2n while tangent and cotangent are periodic functions each with a period.

120 LOVELY PROFESSIONAL UNIVERSITY