Page 127 - DMTH401_REAL ANALYSIS

Unit 9: Functions

9.4.3 Modulus Function Notes

The modulus or the absolute (numerical) value of a real number has already been defined in
Unit 1. Here we define the modulus (absolute value) function as follows:
Let S be a subset of R. A function f : S  R defined by

f(x) = |x|, " x S
is called the modulus function.
In short, it is written as Mod function.

You can easily see the following properties of this function:
(i) The domain of the Modulus function may be a subset of R or the set R itself.
(ii) The range of this function is a subset of the set of non-negative real numbers.
(iii) The Modulus function f : R  R is not an onto function (Check why?).

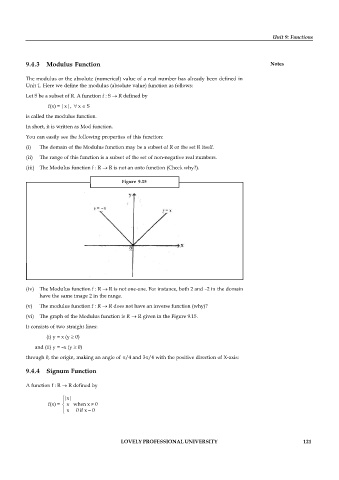
Figure 9.15

(iv) The Modulus function f : R  R is not one-one. For instance, both 2 and –2 in the domain
have the same image 2 in the range.

(v) The modulus function f : R  R does not have an inverse function (why)?
(vi) The graph of the Modulus function is R  R given in the Figure 9.15.
It consists of two straight lines:
(i) y = x (y  0)

and (ii) y = –x (y  0)
through 0, the origin, making an angle of /4 and 3/4 with the positive direction of X-axis:

9.4.4 Signum Function

A function f : R R defined by

ì |x|
ï
f(x) = í x when x  0
ï x 0 if x  0
î

LOVELY PROFESSIONAL UNIVERSITY 121