Page 292 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 23: Developable Surface Fitting to Point Clouds

(2) Two small eigenvalues  ,  but nearly equal coefficients h , h , (|h – h |  ): The Notes
10
1
2
20
10
20
surface D can be well approximated by a cylinder of revolution, compare with (5) in
section 23.3.1. The axis and the radius are computed according to Section 23.3.
(3) One small eigenvalue  and small coefficient h : The surface D is a general cone and its
10
1
vertex is
1
v = (h , h , h ).
h 14 11 12 13
(4) One small eigenvalue  and small coefficients h and h : The surface D is a general
10
1
14
cylinder and its axis is parallel to the vector
a = (h , h , h ).
13
12
11
(5) One small eigenvalue  and small coefficient h : The surface D is a developable of
14
1
constant slope. The tangent planes of D form a constant angle with respect to an axis. The
angle and the axis are found according to formula (3) in section 23.3. An example is
displayed in Figure 23.4.
(6) One small eigenvalue  characterizes a developable surface D whose tangent planes T are
i
1
tangent to a sphere (compare with (4)) in section 23.3
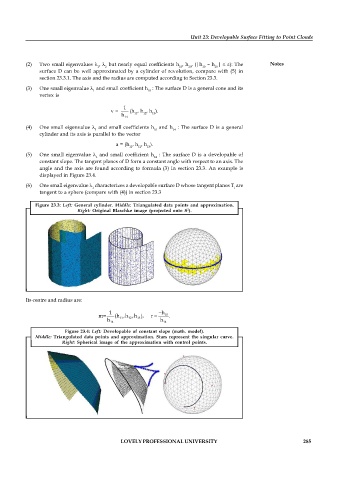
Figure 23.3: Left: General cylinder. Middle: Triangulated data points and approximation.
Right: Original Blaschke image (projected onto S ).
2

Its centre and radius are:

1  h
m= (h ,h ,h ), r  10 .
h 14 11 12 13 h 14
Figure 23.4: Left: Developable of constant slope (math. model).
Middle: Triangulated data points and approximation. Stars represent the singular curve.
Right: Spherical image of the approximation with control points.

LOVELY PROFESSIONAL UNIVERSITY 285